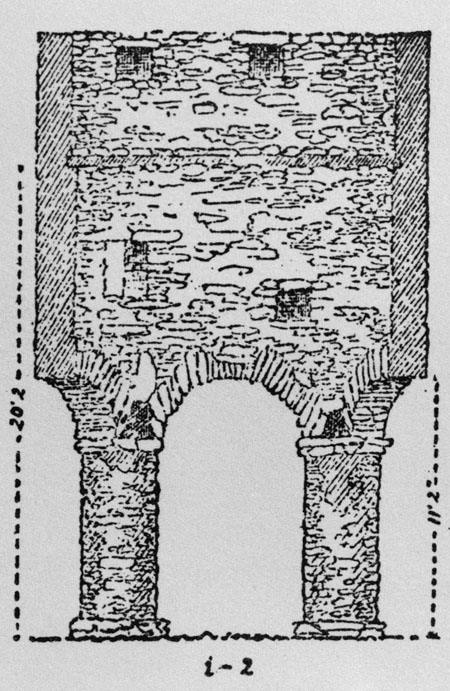
MORE HEIGHT CODES.

Mason measured the distance from the ground to a circuit ridge (undoubtedly for supporting a wooden floor structure) @ 20' 2" or 242". He also measured the distance from the ground to the apex of yet another doorway arch to be 11' 2" or 134".
If the Newport Tower was conceived as a coded structure for preserving old world scientific principles in a far-flung land during the pre-Columbian epoch, , then the circuit ridge line would have been read as residing 243" above ground level, thus preserving highly significant lunar and navigational information.
With the duration of the lunar nutation cycle set to a factorable, calculable duration of 6804-days (6798.36-days actual), ancient astronomers could track the progress of the Moon throughout the cycle as either 243 lunisolar, Sabbatical Calendar months of 28-days each or as 230.4 actually enduring lunar months of 29.53125-days (29 & 17/32nds).
The lunisolar, Sabbatical Calendar tracked the Sun for 7 solar years @ 365.25-days per year (2556.75-days), simultaneously to tracking the Moon for 7.2 lunar years @ 354.375-days per lunar year (2551.5-days) ... for a difference of 5.25-days in the duration of the two periods. Because of this discrepancy, the lunar count was commenced on the 6th day after the solar count had commenced, meaning that both counts would terminate on the same day 7-solar years or 7.2 lunar years later.
The count could proceed in increments of 5.25-days or 126-hours. The ancient Hebrew Reed measurement (10.5' or 2 X 5.25' ... 126") was devised for this count, of which there were 486 periods (243 X 2) of 5.25-days for the lunar count and 467 for the solar count. In terms of full days, the count could, of course, be mainly in increments of 21-days (3-weeks).
Note: It's highly probable that ancient druids or their counterparts had calibrated "staffs" or "rods" of 5.25' (half a Reed) and from that alone could keep track of the daily positions of both Sun & Moon under the Sabbatical Calendar system.
Note also: The ancient Hebrew Reed of 10.5' is 10 Greek feet of 12.6" each. It is also 5 Assyrian cubits (25.2" each) or 1/500th of a Greek mile (5250'), 1/60th of a Greek Stadia (630') or 1/50th of a Short Stadia (525').
The point is, if this height was being interpreted as 243", then an entire tutorial on the Sabbatical Calendar method could have stemmed from that reading.
Mason measures the distance from the ground to the arch centre apex as 11' 2" or 134".
Under the ancient system of highly significant, scientific numbers, this height would make sense as 134.4" and relate directly to the equatorial size of the Earth.
The height would also make sense at several other numbers in very near proximity, ranging from 132, 133.33333, 135, 136.08, etc.
Exploring the merits of 134.4, the following results are obtained:
The sum of 134.4' would equate to 1/45th of 1-minute of arc under the Great Pyramid's literal reading (for navigation purposes) of the equatorial size of the Earth, or 1/2700th of 1-degree of arc.
Alternatively, in the 24883.2 Greek mile equatorial circumference (130636800') there would be 26880 Roman miles of 4860' (13440 X 2).
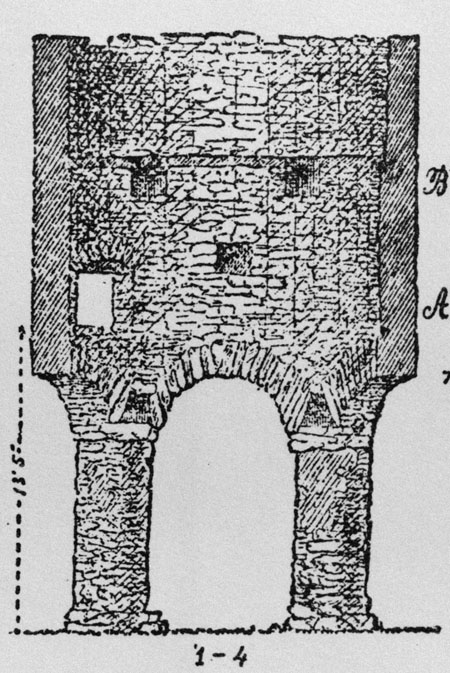
In his final segmentation of the tower, Mason gives a height of 13' 5" (161") from the ground to the sill of a window.
This measurement would make perfect sense @ 161.8034" or 162", as it would then encompass one of the most important mathematical discoveries of antiquity, and something essential to know if the conditions for "civilisation" were to be realised.
The PHI ratio is 1.618034 to 1 and is incorporated copiously into ancient architecture. One such edifice was the Great Pyramid of Egypt, where the surface area of all four sides (including the symbolic capstone area at the top) is in a ratio of 1.618034 to 1 with the area that the pyramid covers over its base.
The existence of such a value on the Newport Tower would certainly lend credence to the assumption that it was pre-Columbian and set up as a repository of the essential codes from which civilisation could be built.
Although the various cousin nations of Europe might have settled to particular national preferences for a "foot", "fusse" or "fot" that varied in length to that of a cousin nation, a close scrutiny of all increments in common usage would show that everything was based upon the same "inch" and that the differing rules of length were in ratio one with another (according to the number family used).
Trade between the nations was made easy in the international marketplaces due to the direct ratio relationships of one nation's preferred, say, Talent weight of grain, with that of another.

By using the PHI formula, ancient assayers found a way to, essentially, "square the circle" and produce highly accurate tubs for dispensing both dry goods and wet goods for sale in ancient marketplaces.
For a king or provincial governor/ priest, trying to keep peace within regional society, few things were more threatening to harmony than "short measures" being meted out in the market place. This problem has required eternal vigilance, worldwide, since time immemorial. The responsibility to know what constitutes a correct measure in weight, volume or length, as well as the enforced maintenance of precise standards, was always a primary function of localised leadership.
In order to make very accurate round tubs for dispensing perfect quantities of grain or other dry & wet goods in the public marketplaces, it was essential to know the PHI formula and more particularly the PHI reciprocal.
The method used was 10" ÷ 1.6180349 = 6.1804".
Capacities for wet and dry goods were in cubic inches (British standard inches... which weren't necessarily British at all, but much, much older in origin) and, as stated, each capacity had to contain scientific information. The number generated by the Egyptian Theban volume (11664 cubic inches) relates to navigation and the sum of 11664' would be 1/11200th of the equatorial circumference. The value was also useful in lunar cycle calculations and, of course, the Roman foot was 11.664".
As another example, the old English bushel (before it drifted ever-so-slightly) was 2160 cubic inches and related to the 2160-years the Sun spends in each of the 12-Houses of the Zodiac during the 25920-year duration of the Precession of the Equinoxes.
So, what is the formula for building a round tub containing exactly 1 bushel of capacity?
The original bushel of England was, undoubtedly, based upon a Sumerian/ Babylonian volume called a Homer, which was 21600 cubic inches. The English Winchester bushel was, originally, 2150.42 cubic inches or very close to 6 X 6 X 60 cubic inches (2160 cubic inches...1/10th of a Homer).
In ancient England the tub created to measure out a correct bushel volume was described as, "any round measure with a plain and even bottom, being 18.5 inches wide throughout and 8 inches deep". This surviving volume from the old English Winchester Standard, undoubtedly, had a pedigree founded upon much older traditions, passed down from distant ancestors.
But the Winchester bushel represents a slightly "drifted" standard, inasmuch as the 2150.42 cubic inch volume is without meaning, whereas a 2160 cubic inch volume would be highly significant. It's plausible to assume that the original coding of the bushel was "lunar", like so many other ancient national standards of the Mediterranean/ European region.
This exact description for a bushel (given above) became the volume of the American bushel, which is 2150.42 cubic inches ...derived from the old Winchester Standard and, thankfully, still preserved in the US.
Using the PHI based rendition mentioned above at 6.18034 inches the true method for making the base and sides of a bushel tub was: 6.18034 X 3 = 18.54102 inches ÷ 2 = 9.27051 (squared) = 85.94235566 X PI = 270 square inches X 8" (the sides of the tub protruding above the base) = 2160 cubic inches...with a margin of error of .033 of a cubic inch.
So it's easy to see that The Old English bushel @ 2160 cubic inches was, by pedigree, simply 1/10th of a Babylonian Homer (21600 cubic inches).
Use of the PHI reciprocal, converting to 6.18034", ensured that all round measuring tubs, fabricated for use in all the marketplaces of the inter-trading nations, were very accurate and true measures of the quantities to be dispensed. There was no excuse for "short-measures".
Similarly, standard weights for assaying were first based upon counting out grains of wheat from a healthy crop. The number of grains had to equal one of the numbers from the special parcel of scientific values. Such carefully configured "official" or "master" weights were then used to fashion duplicate sets for the market-places.
The point is, all of the measurement standards by which the pyramids were built are preserved in the oldest standards of the European nations and were carried abroad by them in their explorations and colonisations of other continents in antiquity. These ages-old standards were maintained and preserved by the European nations for the past 7000-years or so.
HERE ARE SOME COMPARISONS OF KNOWN ANCIENT VOLUMES WITH THE (corrected) ENGLISH-AMERICAN BUSHEL.
Question: So how do we achieve this degree of accuracy with a round marketplace tub?
Answer: We have to make the circular base diameter of the tub according to increments (increases) of 6.18034"
The average young, visually acute carpenter can work to tolerances of 1/32nd of an inch and some to 1/64th ... (but sadly, not this old carpenter anymore).
In the fabrication of a bushel tub, the carpenter, laying out the diameter of the circular base, needed only to achieve about 6 & 2/11ths" per segment to ensure good relative accuracy in the final cubic inch capacity of the tub.