At the very heart of Stonehenge's geometry are four marked positions called the "station stones". There was,
anciently, yet another position, long since eradicated, slightly to the north of the Heel Stone, which seems to have been directly related to the 4 station stones.
There have been several theories about the purpose of the station positions, but few effectively explore the surveying aligned geometry by which the stations were conceived and accurately placed.
Indeed, it is out of an understanding of the intended geometry in the station positions that we can begin to fathom the minds of Stonehenge's architects, as well as the approach they took to laying out the observatory.
Let's unravel the tangled cobweb of "station stone" codes strand by strand and layer by layer:
The first thing we have to discuss is the "orientation" of Stonehenge and the seemingly strange angle, off North, at which the station stones rectangle reposes. Why does the rectangle lie on that angle, with the 4 stations at or near 342, 296, 162 & 115-degrees respectively?
Also the midsummer sunrise appears to be at 90-degrees to the line running from the mound station (stone number 94) at 342-degrees, to the station stone at or near 115-degrees (stone number 91)...is this significant?
Next, what do we know about these ancient astronomer/ mathematicians...what kind of information did they value and wish to encode?
We now know the majority of the numbers that they used in the implementation of their art, so doesn't it make sense to sift through those number codes for clues related to the station positions?
We also know that the ancient "Masters of the Craft" were sticklers for tradition and followed known, universal systems that replicated usage of the selfsame numbers from generation to generation.
"The knowledge" had to be religiously preserved and passed on untainted to progenitors. This would suggest that there not be any confusing innovation that deviated markedly from the norm and that all parts of standing stone circle sites remain easily decipherable throughout ensuing generations...based upon a standard set of numbers.
Figure 27: Knowing
the traditional approach taken, coded numbers used and general modus operandi
of the ancient astronomer/ mathematicians, aids us in deciphering what they
intended to convey via the "station stones" layout. There are many codes related
to the station stones rectangle, its orientation, its dimensions and the geometry
it supports, from the Sarsen Circle to the near vicinity of Heel Stone. THE CODES OF POSITION. (1). Stone 91 resides
at 115-degrees azimuth, approximately. Given the width of the fallen stone,
that degree angle falls into a range of azimuth between 114.8 to 116.8-degrees,
with an average of around 115.8-degrees. (2). If we go 180-degrees
opposed to the 11.52 - 11.57-degree position, then we are in the vicinity
of stone 93. Technically, this should put the stone at 29.52 - 29.57-degrees,
but the stone lies slightly north of that mark by about another degree. Of
the 4 station stones, this one proves to cause a slight irregularity in the
geometry of the station stone rectangle and one could easily surmise that
it has been pushed or rolled to the north at some time in history. The distance
between it and stone 94 could, however, have been deliberately "shortened
up" to incorporate another code...a distance of 112 feet, instead of the expected
113.4 feet, looks plausible. (3). Returning to the
eastern side of the site, station stone 92 sits on a mound and complies nicely
to 162-degrees. This is a very clear-cut and sensible code for "rounded PHI"
or pure PHI (1.6180339). The ancient measurement system, in many sexagesimal
applications, is resolvable by 162. The Great Pyramid base dimensions, when
divided into 1.62 increments, provides the number sets upon which the British
system of "weights" was devised. Number sets within the British standard measurements
were founded on 162 as a base number and there was a second set of measurements
based upon pure PHI, such as the Megalithic Yard (32.360678 inches true...1.6180339"
X 20). (4). Going 180-degrees
opposed to the 162-degree position is station stone 94, which resides nicely
on a mound at 342-degrees. But why 342-degrees...what merit does such a degree
angle have as a surveying benchmark or locating position? Well, let's see...342-degrees
is exactly 18 degrees short of true north (360-degrees). It's indicative of
dividing a circle up into 20 parts, with station stone 92 (@ 162-degrees)
representing the 9th increment and station stone 94 representing the 19th
increment...but what's the relationship, in degrees of arc, between stone
94 (324-degrees) and stone 91 (115.2-degrees)? (5). This is where it
starts to get very interesting and where the genius of the ancient architects
is revealed. The whole business of establishing a surveying line between 324-degrees
and 115.2-degrees had to do with a 90-degrees opposed, central fix onto the
rising sun, as it made its appearance on midsummer's day (summer solstice).
Everything had to work to known numerical codes and here's the apparent formula: (a). The degree of arc
difference between 324-degrees & 115.2-degrees is 133.2-degrees. (b). Half of 133,2-degrees
is 66.6-degrees. (c). The sun's point
of rise, above the north-eastern horizon, was calculated to be 66.6 degrees
around to the east of either stone 91 or stone 94. This places the sun's true
calibrated azimuth angle, for time fix observation, at 48.6-degrees azimuth. Figure 28: The summer
solstice sunrise, as viewed at Stonehenge. Many authors are under the illusion
that the event occurs over the Heel Stone. In fact the sun is quite elevated
when it traverses the Heel Stone position and has already made its initial
appearance a couple of degrees to the north. The above picture is based upon
Prof. John North's depiction of the event at Stonehenge (see Fig. 183, pg.
467, Stonehenge, Neolithic Man And The Cosmos). The red outline shows how
the Slaughter Stone, when standing, would have blocked the view to the Heel
Stone, as observed from site centre. It's of interest to note
that there is a "missing stone" position (stone 97) that once sat to the left
and beyond the Heel Stone. Perhaps its size and position once marked the true
point of midsummer sunrise. Did it once comply with a 48.6-degree azimuth
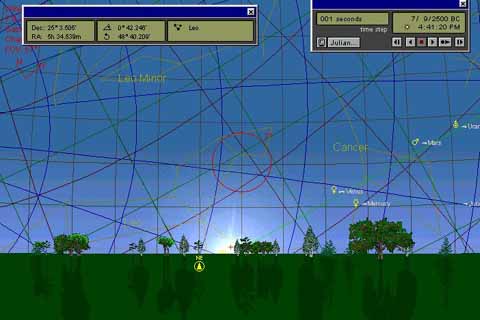
angle? Figure 29: The picture
shown depicts a summer solstice sunrise, as observed from Stonehenge in 2500BC.
The dark foreground shown indicates where the sun would rise were the horizon
level with the observer (like from a beach, looking toward the sea horizon).
A red cross depicts the approximate level of the horizon as seen from Stonehenge,
simultaneously to the azimuth angle of 48.6-degrees. The evidence would suggest
that "first glint" from the sun's fiery corona was observable from very close
to 48.6-degrees and that this was the most extreme northern azimuth angle
from which "first glint" could be observed at Stonehenge. Prof. John North
calculates first glint of the upper limb of the summer solstice sun (circa
2500BC) to break upon the Stonehenge horizon at 49.37-degrees...another .77-degrees
further south of 48.6-degrees. WHAT
OTHER CODE BEARING EVIDENCE INDICATES 48.6-DEGREES AS THE SURVEYOR'S BENCHMARK
AZIMUTH FOR THE ERECTION OF STONEHENGE? Quite a lot actually!
The process of finding 48.6-degrees off north is based upon the arc difference
between 342-degrees & 115.2 (both excellent numbers) to attain a 3rd dynamic
number...66.6-degrees...this being the mid-arc value. There are several more
codes in the pipeline, which support the choice of 48.6-degrees as the chosen
benchmark/ summer solstice azimuth. One compelling piece of evidence relates
to the nature of 48.6 itself. THE STATION STONES
GEOMETRIC CODES AND 48.6-DEGREE ORIENTATION. This next phase of code
extraction might prove to be a little ponderous and involved, as there is
a lot of geometry to identify. Each linear or circular feature of the geometry
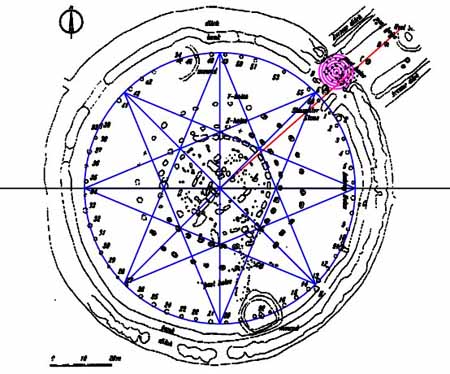
is also coded to the "most used" values of antiquity. To begin: Figure 30: An 8-pointed
star is placed upon the Stonehenge site with ray points extending to the Aubrey
Circle (288 feet diameter). Ray-points reside upon the primary and secondary
points of the compass. Simultaneously to this a fix is taken from site centre
onto the "first glint", horizon position of the rising sun and it is determined
that the dynamic code, 48.6-degrees can be used to very adequately describe
this annual occurrence. The star geometry will now be adjusted to suit this
close proximity midsummer sunrise vector, which involves rotating the star
26.1-degrees clockwise. Figure 31: A centre
section of the star, between ray points, now resides upon 48.6-degrees and
this azimuth angle provides the viewing alignment to the summer solstice sunrise.
Note how 4 ray-points of the star now fall onto or extremely close to the
station stone positions. The central rectangle of the 8-pointed star, which
falls to those positions, will be retained and then very marginally adjusted
for the extraction of additional codes. Note also the central magenta circle,
which is two PHI reductions of the Aubrey Circle (288 ( PHI ( PHI = 110.00
feet). This diameter describes what the PHI based, symbolic, coded outer diameter
of the Sarsen Circle was, however the actual, physical Sarsen Circle structure
was manipulated into an elliptical shape to build in additional codes. We
will now discuss why this was done and the coded values that were being sought
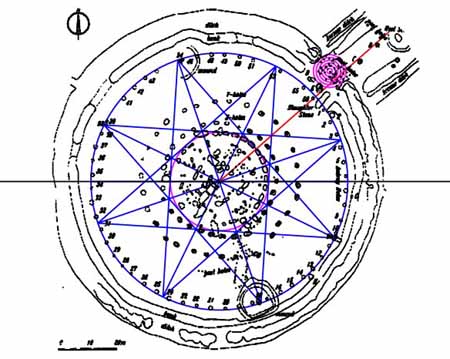
after. Figure 32: This is
a very important picture, the significance of which leads to an understanding
of the mathematical template, which formed both the station stone rectangle
and the "elliptical" extremity positions of the Sarsen Circle. Consider the
following carefully: (1). When an 8-pointed
star pattern is placed perfectly within the confines of the Aubrey Circle,
its centre square would, with only minor error, contain the Sarsen Circle...if
the Sarsen Circle was configured to be a perfect circle. In such a case the
Sarsen Circle's outer diameter would be 2 PHI reductions of the Aubrey Circle. (2). The central rectangles
of the 8-pointed star would measure 265.94 feet long X 110.2 feet wide, but
the ancient astronomers decided to manipulate this slightly in order to include
or extract two additional, very useful codes. The intended size of the station
stones rectangle was 264 feet long & 113.4 feet wide. (3). With this small
adjustment to the 8-pointed stars central rectangle, the Sarsen circle was
made elliptical, such that its outer limit, facing toward 45 - 48.6-degrees
azimuth through 225 - 228.6-degrees azimuth, achieved 113.4 feet of diameter.
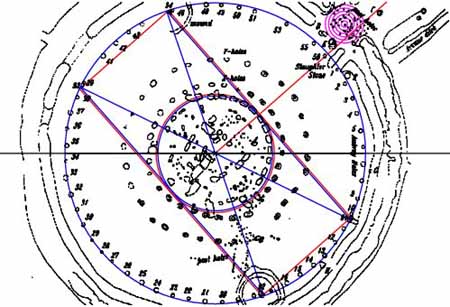
Let's see how this truly relates to the existing ruins of the Sarsen Circle: Figure 33: Although
little symmetry remains of the present day Sarsen Circle at the 225 - 228.6-degree
end (SW), the structure remains in measurable condition toward 45 - 48.6-degrees
(NE). Two sets of vectors or circles are shown herein. The blue set relates
to the dimensions achieved by internal geometry of an 8-pointed star placed
within the confines of the Aubrey Circle. The red set is based upon a marginal
adjustment of the central rectangle of the 8-pointed star. Note how the red
circle, as well as the red rectangle's facing line brush the outer edge extremity
of the Sarsen Circle at 45 - 48.6-degrees azimuth. This is the template that
led to the creation of an elliptical Sarsen Circle, which was 113.4 feet in
diameter through this azimuth angle. The question must therefore be asked...why
was it necessary to code 113.4 feet on the main structure of Stonehenge? 
Two very important numbers spring to mind, which were dynamic codes. The first
and foremost is 115.2, which in various volumes, ranging from 1.152 to 11520
or greater, was incorporated into all major sites.
It is logical to conclude that stone 91 included 115.2-degrees as the primary
code in its intended azimuth reading. A second, lesser used, code relates
to placing a 14-pointed star onto the Aubrey Circle, with one ray on east
and another on west (equinox line). Such a star pattern perfectly fits into
place at Stonehenge and was used for "Sabbatical Calendar" calculations. Station
stone 91 relates to a ray of that star pattern, falling on 115.7142857-degrees
azimuth.
There are "checks and balances" in the station stones geometry, which would
suggest that this stone should reside (centre) at 295.2-degrees but also represent
295.3125-degrees (to code the ancient number for the lunar month...29.53125-days).
It is tantalisingly close to where it should be, theoretically. We'll leave
it for the moment, weigh up the totality of factors leading to an understanding
of the "station stone" geometry and codes, then return to stone 93 later.
This should describe conditions at Stonehenge reasonably well, as the midsummer
sun rises on a severe diagonal angle a couple of degrees north of the Heel
Stone.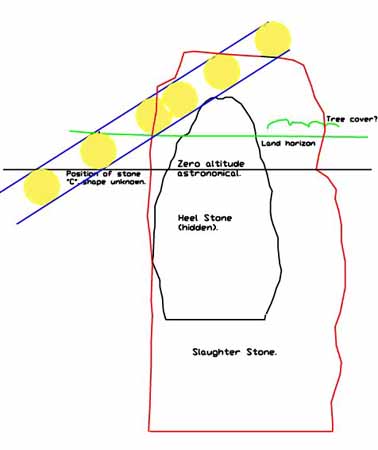
It is this type of approach that ancient astronomer/ mathematicians would
have taken, such that marked, measurable positions could always provide a
key for renewed decipherment of forgotten principles. From the pyramids to
Stonehenge, the archaic structures themselves fulfilled the role of eternal
teachers. Beyond war, plague and the disruptive, uncertain circumstances of
mortality, the stone repositories of humanity's most cherished knowledge retained
the ancient codes, safe and intact for future, enlightened generations.
This is a strong lunar code, which might account for choosing it as an easily
remembered surveying benchmark.
With the 18.613-year, nutation cycle of the moon using a calibration of 6804-days
to describe it, the sum of 48.6-days = 1/140th of the period. We know that
this 18.613-year period endured for 243 months (of 28 days each) and 48.6
is 1/5th of 243.