

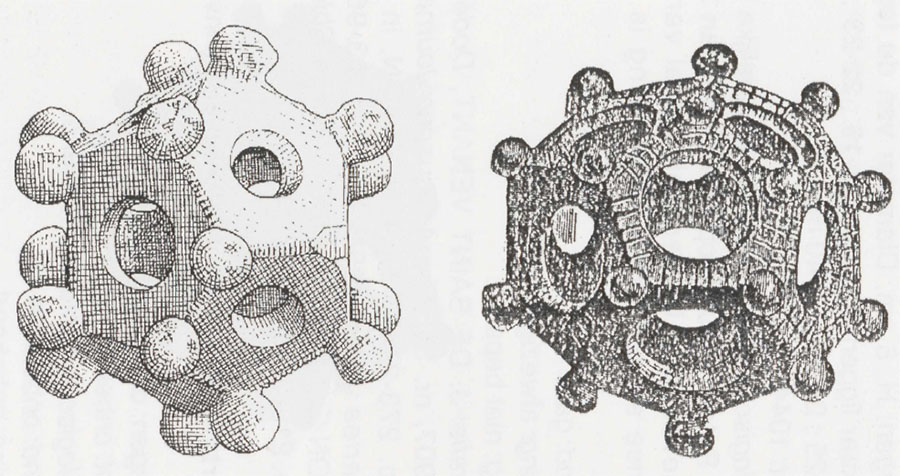
These bronze artefacts are so-called Roman dodecahedra (plural of dodecahedron) of which about 120 have been found in the Celtic countries of Europe. Strangely, none of these have been found in Italy, home turf of the Romans. To the left is seen a dodecahedron from the Römermuseum Schwarzenacker, Homburg, Germany collection. To the right is seen another pristine example from the Hunt Museum in Limerick Ireland

To the left is an Icosahedron from Archaeological Service Canton Aargau, Brugg, Switzerland (Vindonissa Museum). To the right is a dodecahedron from the Gallo-Roman Museum, Tongeren, Belgium.
The basic design of these artefacts remains relatively constant (12 pentagonal faces with knop-shaped legs), however, there can be a range of different incised designs in the layout of the faces.
The actual function of these artefacts cannot be explained by our historians or archaeologists, although many theories abound, none of which seem particularly convincing. Also, there is no mention of these items in old historical records of the Romans or those of anyone else for that matter. They are such an enigma that academics have pretty-much come to the defeatist conclusion that "we will never know what these dodecahedrons were used for".
In reviewing the academic literature, one begins to see why the mystery will probably never be solved within that community. Here are some of the impediments and pitfalls our experts create for themselves:
All archaeological measurements are given in metric increments (centimetres or millimetres), which is a modern system that was created as much as 2000-years after some of these dodecahedra were cast in bronze. Earlier exemplars in stone or wood could predate the latter, more refined ones, by yet another thousand years or more. This metric measurement preoccupation renders the encoded numbers invisible or unrecognisable. Our academics know a large swathe of the ancient measurements that were in use two thousand years ago or before, so why not apply them to this study?
Most assuredly, the various sized holes in the 12-faces, the concentric circles that circumnavigate them, as well as the additional incised lines or dotted circles, etc, infer that there is something vividly measurable going on and it's evident that each hole or ring has been very purposely fabricated to represent a known, precise and sought-after measurement.
A number of dodecahedra have calibration marks along their edges or around the holes in their faces. To the left is seen a calibrated dodecahedron from Hereford Museum, Kenchester and to the right is seen another from Goodrich Castle. Yet another one found in Wales shows etched calibration marks.
In their reports, archaeologists will often round out measurements of the holes in the dodecahedron faces to the nearest millimetre only, which is grossly insufficient. It's much appreciated by researchers when measurements can be supplied to 1/10th of a millimetre, but it is nigh on impossible to acquire more refined measurements undertaken with electronic vernier callipers. Precise measurements and scaling must precede any serious analysis of these artefacts, but our experts don't seem to think there would be anything significant to find anyway, so don't subject the dodecahedra to rigorous measurement analysis.
Despite the fact that 25.4 millimetres (1 British Standard Inch) or fraction expressions of the same will recur repeatedly in the faces of dodecahedra, no-one seems to have arrived at the conclusion that the enigmatic "inch" recurrence is worthy of serious investigation.
Almost anything considered high-art, technically advanced or an engineering feat within ancient European civilisations seems to be automatically attributed to the Romans, which is certainly the case for these dodecahedra artefacts. This is a hangover of biased, classicist-isolationist historical interpretations of European history where, first came the Roman conquerors, who finally retreated after centuries of domination, only to be replaced by Rome's State church. With the pedigree of the church being Greco-Roman it was in their interests to push the concept that the Roman armies had found everything in the regions they invaded to be backwards, rudimentary or crude until Rome delivered the great-unwashed masses out of their depravity and into enlightenment and high-civilisation.
This general process of misattribution further hobbles or seriously limits proper investigation into the purpose and function of dodecahedra artefacts, inasmuch as focus is directed, almost exclusively, to what Roman armies might have used them for as range-finders or other battle related paraphernalia, including club-heads. The druids or others don't get any serious consideration, despite the fact that they are historically recorded as having been advanced mathematicians and astronomers.
Within controlled academia the ability to do open-ended, ground-breaking research can be severely curtailed by the requirements of "peer review" by colleagues. This is largely a racket that ensures one doesn't move too far from the protected consensus opinion upon which academic reputations are built and anyone venturing too far out into left field is considered a maverick who threatens the insulated central body.
By the same token academia, these days, is lumbered with deep-set social responsibilities and crippling political agendas. When it comes to European history, especially, the requirement is to be self-effacing, while lauding and applauding the (often meager) accomplishments of other ethnicities. Under guises of political correctness and racial sensitivity, the door is opened for antagonistic non-European, prejudiced individuals, to write and interpret our history for us, while pushing their own cultural-Marxist or similar wheel-barrows. All one has to do is sit through lectures in our western universities to see just how far out of kilter the abysmal problem of European history-misrepresentation has become.
But, with regards to the dodecahedra artefacts,what one person can build, another can generally duplicate or back-engineer, sufficient to make sense of what's going on.
So, let's cut to the chase, bypass all the confusion of modern-day naysayers and source testimony directly from an observer who lived contemporary to the druids, before they fell under Roman domination
Julius Caesar, who was a very thorough historian, writes the following regarding the late era druids of his time (circa 55 BC) and practices within their many universities in Britain, where students from Gaul and elsewhere, including Rome, went for training:
'They hold aloof from war and do not pay war taxes; they are excused from military service and exempt from all liabilities. Tempted by these great advantages, many young men assemble of their own motion to receive their training, many are sent by parents and relatives. Report says that in the schools of the Druids they learn by heart a great number of verses, and therefore some persons remain twenty years under training'.
'They do not think it proper to commit these utterances to writing, although in all other matters and in their public and private accounts they make use of Greek characters. I believe that they have adopted the practice for two reasons- that they do not wish the rule to become common property, nor those who learn the rule to rely on writing and so neglect the cultivation of memory; and, in fact, it does usually happen that the assistance of writing tends to relax the diligence of the student and the action of memory...They also lecture on the stars in their motion, the magnitude of the Earth and its divisions, on natural history, on the power and government of God; and instruct the youth in these subjects' (see De Ballo Gallico, VII, 15, 16.).
Historian, Isabel Hill Elder writes, 'The students at these colleges numbered at times sixty thousand of the youth and young nobility of Britain and Gaul. Caesar comments on the fact that the Gauls sent their youth to Britain to be educated...It required twenty years to master the complete circle of Druidic knowledge. Natural philosophy, astronomy, mathematics, geometry, medicine, jurisprudence, poetry and oratory were all proposed and taught-natural philosophy and astronomy with severe exactitude' (Elder refers to Strabo I IV, page 197. Caesars Comm. Lib V. Sueotonius, V Calegula. E. Campion, Accounts of Ireland, pg. 18.).
Isabel Hill Elder further writes, 'The education system adopted by the Druids is traced to about 1800 BC when Hu Gardarn Hysicion (Isaacson), or Hu the Mighty, led the first colony of Cymri into Britain from Defrobane, where Constantinople now stands'.
Further commenting on Hu Gardarn Hysicion, Isabel Hill Elder writes that he, 'is commemorated in Welsh archaeology as having made poetry the vehicle of memory'. Elsewhere she writes, he 'is said to have mnemonically systematized the wisdom of the ancients...'. She goes on to say, 'The published compositions of the Druids and Bards form but a very small portion of the extant remains of their works. The Myvyrian MSS. alone, now in the British Museum, amount to 47 volumes of poetry, in 1600 pages, besides about 2000 epigrammatic stanzas. Also in the same collection are 53 volumes of prose, in about 15,300 pages, containing many curious documents on various subjects...' (see Celt, Druid and Culdee, pages 54 & 55).
With the testimony of Julius Caesar ringing in our ears, lets see how the druids would have used the dodecahedron artefacts as "memory"devices for mathematically encoding astronomical cycles, ("the stars in their motion"), navigation systems ("the magnitude of the Earth and its divisions"), ... "astronomy, mathematics, geometry" ... "and astronomy with severe exactitude".
THE 1987 DISCOVERY BY BRIAN CAMPBELL, ROMFORD ENGLAND.

A pristine dodecahedron found by Mr. Brian Campbell while digging in his English garden (circa 1987).
Brian Campbell writes:
"Some 20 years ago while digging in my rear garden north of Romford, England I came across a Roman Dodecahedra. At the time I had no idea what I had found until some years later.
I saw one displayed in a Roman Fort Museum outside Frankfurt, Germany.
|
I subsequently took the item to the British Museum, where I was told it was either a fake or one of the best examples of a Roman Dodecahedra found to date. I, of course, know it to be genuine in as much as I discovered it when removing a tree stump and buried perhaps two feet down.
...I have to say I was very disappointed by the response I had from British Museum staff, although to be fair they were working in temporary accommodation during renovations to the Museum when I visited them.
I have always wondered about the little ‘mushroom’ pegs or legs, as they appear to me to be important, not just left over from casting the metal. My theory before I knew the artefact to be Roman was that it was a plumber’s form for checking the diameter of pipes.
Each hole/bore is a different diameter, ten are beautifully formed, each with three concentric circles furrowed around them, while the remaining two, which might be described as top and bottom, are more roughly formed, with no concentric circles.
The bottom/base one in my orientation being the largest diameter bore, the top one being slightly smaller in diameter, but both larger in diameter than any of the ten others. I believe for this reason some have speculated the dodecahedra might have been impaled upon a stake, pike or staff.
Returning to the pegs, which appear to have been purposely shaped into ‘mushrooms’, I can see in my minds eye it covered in a leather jacket, the pegs being pressed through eyelets to hold the covering in place.
Maybe fanciful on my part as the dodecahedra is truly a lovely piece of art-form, functional or not. .. My Dodecahedra is made of bronze I believe and of a brown coppery colour, it truly is magical and fascinating!
I have read that these Dodecahedra may be Druidic in origin and seized by the Romans during their occupations. I find this difficult to believe. For if their value to the Druids was as important as has been speculated, I would have expected the Druids to have secreted them away and at least some to have been found in places other than those occupied by the Roman Legions.
That said, there remains the question why Dodecahedra have not to my knowledge been discovered in Italy, even if only taken there as spoils of war".
BRIAN CAMPBELL SUPPLIES HIGH RESOLUTION, SQUARE-ON-FACE PHOTOS.
After making inquiries through the Internet, I was able to track down Brian and ask him if he could supply high resolution photos and measurements of his dodecahedron, such that I could scale them in AutoCAD and do accurate measurements of the holes and concentric circle rings. For this exercise I would need square-on-the-face images of all 12-faces and also measurements of the holes to be undertaken with vernier callipers. This way, diameters could be made to a couple of decimal points of accuracy, rather to the nearest millimetre or 1/10th of a millimetre that one finds in archaeological reports.
Brian, very graciously, undertook the mammoth task, photographing all 12-faces twice, the first time showing no scale bar, but vernier calliper measurements of the holes for North-South, then East-West. I had asked him to do this, as the the forebears of the druids often laid out their standing stone circles, not as perfect circles, but slightly elliptical. By this means they were able to get two coded circle diameters on each circle by achieving slightly different cross measurements 90-degrees opposed.
This observation related to elliptical circles was made by Scottish engineer, Professor Alexander Thom who undertook measurements and surveys of hundreds of British and French archaeological sites.
Brian then went on to supply a second set of photos showing all 12 faces square-on, but this time with an English 5 pence coin inside the face holes at the level of the face. Knowing that the English 5 pence coin was fabricated to be exactly 18 millimetres in diameter, scaling in autoCAD architectural program could be completed at a very high degree of accuracy.
For the benefit of mainstream archaeologists, all measurements that follow herein will first be rendered in millimetres, then converted to inches and decimal fractions thereof. As this process unfolds the true numerical values that druidic savants memorised, to encode their navigational and cyclic astronomical sciences, will be revealed.
I consider myself to be amply qualified to undertake this analysis, having studied Weights, Measures & Volume standards of the ancient Mediterranean, Continental Europe & Britain for the past 20-years.
WHY DID THE DRUIDS WANT TO ENCODE NUMBERS & HOW DID THE DODECAHENDRON NUMBER-GENERATOR WORK?
The number encoded into the diameter of a circular hole or ring represented the beginning point value or root number, which then doubled, quadrupled, octupled, etc., creating a mathematical progression that built from small numbers (decimal fractions) to potentially huge, whole numbers. By this means a fraction of an inch could ultimately generate a final value that described the equatorial circumference of the Earth in miles.
We use similar methods today to describe such things as the RAM (Random Access Memory) in computers. Remember how they've moved from 1, 2, 4, 8, 16, 32, 64, 128 bits of memory over the years? The dodecahedron process is somewhat similar, except that the number string only increases by doubling the root value and adding it to the ever increasing value.
Much the same method is applied to measuring increases of picture resolution on computer screens in pixels, rising hertz oscillation frequencies, decibels of noise, etc.
With a dodecahedron mnemonic device, a druidic scientist could measure the diameter size of a hole or ring to recall the special root value, then mentally or otherwise recount a whole string of number increases that related to, say, their navigation sciences, the Precession of the Equinoxes, the lunisolar Sabbatical calendar counts or the lunar nutation cycle, etc.
The known system used by the Greeks for their overland measurements was a foot of 12.6", a reed measurement of 10 Greek feet (126" or 10.5 English feet of 12 English inches), a short stadia of 525' (or 500 Greek feet), a long stadia of 630' (or 600 Greek feet), a mile of 5250' (or 5000 Greek feet). All Greek overland measurement were divisible by either "6" or "7", as was the ancient Irish mile of 6720'.
From a general overview of the metrology systems used by the Romans or ancient people of Britain, we can deduce that the Greeks also used increments of 5.25' and 6.3'.
The Romans had a foot of 11.664"*. Their pace measurement was 5 Roman feet or 58.32" (4.86'). Their mile was 5000 Roman feet, which would be equivalent to 4860 ancient or modern English feet. The Roman measurements were divisible by 6.
The ancient English had a foot of 12", a yard of 3', a fathom that was originally 5.5', a rod or perch that was 16.5' (3 fathoms), a chain of 66', a furlong or furrowlong that was 660', a mile of 5280' and a league that was 16500' (3.125 miles). The English overland measurements were divisible by the number 11, as were the ancient Scottish measurements, with their mile of 5940' (1 furlong greater than the English mile).
It is universally accepted that we use the ancient chronology system of the Sumerians, with 60 seconds in a minute, 60 minutes in an hour and 24-hours in a day, etc. Likewise, our experts accept that the 360-degree compass system, breaking down to 60-minutes per degree of arc and 60 seconds per minute of arc, also came from the Sumerians. The question therefore goes begging: Why didn't we inherit the Sumerian Weights, Measures and Volumes systems as well?
The fact of the matter is that WE DID and all of the Great Mediterranean, Continental European and British Isles civilisations shared an integrated metrology system, based upon the same numbers and the same "inch". Although individual national cubits, standard volumes and grain weights could vary across borders, they were all in ratio with the standards of surrounding cousin nations.
These cousin civilisations, situated all around the Mediterranean and Europe, encoded their sciences of navigation, cyclic astronomy, and lunisolar calendar systems into numbers that could be memorised and the special parcel of numbers formed the basis of all their Weights, Measures & Volume standards.
*The Roman foot was found to be 11.664 inches by John Greaves, professor of geometry, who in 1639 went to Rome specifically to ascertain the length of an ancient Roman foot. Greaves located a monument of Roman architect, Stalius Asper and measured bas relief instruments used by him in the first century A. D. Greaves concluded, after careful investigation, that the Roman foot, 'contained 1944 such parts as the English foot contains 2000'. This means a Roman foot of 11.664 inches (11 & 83/125ths).
FACE 1

This is the first hole, which Brian Campbell measured with vernier callipers to be 22.31 mm East-West and 22.46 mm North-South. Using Brian's measurements, the photo was taken into the exacting confines of AutoCAD and scaled to the dimensions he gave.
The East-West measurement would equate to 0.87834646". The intended figure for the slightly elliptical circle opening was .875" or 7/8ths of an inch. The error is only about 1/300th of an inch and undetectable to the human eye.
From this root value in a whole number decimal fraction the druidic scientist would create the following mathematical progression:
.875. 1.75, 2.265, 3.5, 4.375, 5.25, 6.125, 7, 7.875, 8.75, 9.625, 10.5, 11.375, 12.25, 13.125, 14, 14.875, 15.75, 16.625, 17.5, 18.375, 19.25, 20.125, 21, 21.875, 22.75, 23.625, 24.5, 25.375,
26.25, 28, etc.
The continuing string produces such values as 315, 350, 420, 472.5, 490, 525, 560, 630, 700, etc.
This mathematical progression provided the druidic savant with values required for reading lunar cycle durations, as well as navigation under the so-called Greek system of overland measurements. For example, the sum of 472.5-days would equate to 16 lunar months of 29.53125-days each (29 & 17/32nds). This value for a lunar month is correct to under one minute of time.
Likewise, the sums of 2.265', 5.25' & 10.5' would be quarter, half and full expressions of the Greek/Hebrew reed measurement (10 Greek feet or 10.5 English feet). The sum of 525' would be a Greek short stadia, 630' a Greek long stadia and 5250' a Greek mile of 5000 Greek feet @ 12.6" each.
The progression continues to grow until the number 130636800' is achieved. This equates to 12 X 12 X 12 X 12 X1.2 Greek miles of 5250' each or 24883.2 such miles to describe the equatorial circumference of the Earth.
But Mr. Campbell measured the North-South diameter of the hole to be 22.46 mm, which equates to 0.88425197".
The intended diameter was .88" or 22/25ths of an inch, and the error is only about 1/100th of an inch overflow, or beyond visual detection.
From this root value reading in decimal fractions the druidic savant would create the following mathematical progression:
.88, 1.76, 2.64, 3.52, 4.4, 5.28, 6.16, 7.04, 7.92, 8.8, 9.68, 10.56, 11.44, 12.32, 13.2, 14.08, 14.96, 15.84, 16.72, 17.6, 18.48, 19.36, 20.24, 21.12, 22, 22.88, 23.76, 24.64, 25.52, 26.4, etc.
The progression increases to generate values like 66, 660, 5280 7920, all values that form the "11" navigational system with a mile of 5280'. It continues through to 130680000' or 24750-miles of 5280 feet, the value used to describe the equatorial circumference under the English mile navigational system.
The sum of 1760' was 1/3rd of a mile, 2640' was 1/2 a mile, 1056' was 1/4th of a mile, 7920-miles was the diameter of the Earth under this navigational system and 7920 X 3.125 = 24750, which was the value used to describe the equatorial circumference of the Earth when navigating by the 5280' English mile, 5940' Scottish mile or 16500' English league (3.125-miles).
Essentially, this top hole in Brian Campbell's dodecahedron provides beginning values for two much-used navigational systems which are encoded into the dimensions of the Great Pyramid of Egypt. One of these worked by using a combination of the 6&7 number families, whereas the other used the 11 number family.
The 6&7 method is based upon the literal base measurement of the Great Pyramid, which was 756' so-called British standard feet (of 12-inches) per side length when the pyramid was first built, obviously, as a Bureau of Standards. Thousands of years of time and sand-blasting erosion have, perhaps, slightly diminished that reading by an inch or two, but the intended length to represent the beginning point of a mathematical progression (related to the equatorial circumference of the world) was 756'.

Sir William Flinders Petrie deduced that the "inch" used on the Great Pyramid was within 1/100th of an inch (visually undetectable) to the British Imperial Inch. Other researchers had come to similar conclusions, one of which put the Pyramid Inch to within 1/1000th of the Imperial Inch.
The matter related to the precise length of either the true inch or intended, final length of the Great Pyramid can be calculated mathematically by using the Egyptian Royal cubit, one of which is in the Turin Museum and has a length of 20.618 British Imperial inches (refined to the 3rd decimal point). The obvious intention of the architects-mathematicians-builders was to make the base length of the pyramid comply to 440 Egyptian Royal cubits or 756' (9072"). This was based upon a royal cubit of 20.61818182" or 20 & 11/68ths-inches.
The archaeological evidence shows conclusively that there were, in fact, 3 different length Egyptian Royal cubits (20.61818182", 20.625" and 20.736"*) and no known examples were graduated for measurement use by a builder. The overall length of each was designed to encode the equatorial circumference of the Earth under 3 separate navigational systems.
Under the literal 756' side length coding, two full circumnavigations of the Great pyramid equated to 6048' and this represented 1-minute of equatorial arc ... X 60 = 362880' for 1-degree of arc ... X 360 = 130636800' for the full circumference. This finalised value in English feet of 12" each equals 12 X 12 X 12 X 12 X 1.2 Greek miles of 5250' each (24883.2-Greek miles). This is also 76032000 Egyptian Royal cubits of 20.61818182".
A simpler way was to use the Egyptian Royal cubit as a mnemonic device, mentally accepting that the length represented "miles" of 5280'. Therefore, 20.61818182 X 1200 = 24741.81818181-English miles or 24883.2-Greek miles.
In fact, the Egyptian Royal cubit, with its variable marked segments did exactly the same thing as the druidic dodecahedron holes, in providing values for beginning mathematical progressions.
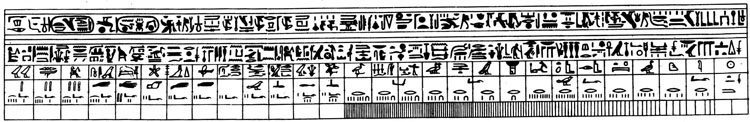
Due to the fact that the segmentation of the Egyptian Royal Cubit is by no means even, it could not be used by a builder in the normal trade practices of measuring and cutting materials.
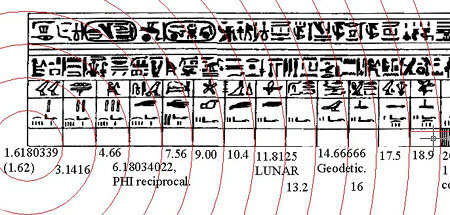
The true purpose of the segmentation was to provide beginning numbers for mathematical progressions, a PHI inch, the PHI reciprocal for fabrication of precise volume tubs for the market place, an inch X PI, as well as lunar and navigational values. It acted in the same capacity as the latter era druidic dodecahedra mnemonic devices to generate numbers.
So, under the navigational method encoded into the true base measurement of the Great Pyramid, using so-called Greek miles, all of the Greek measurements fit the 756' length of the Great Pyramid, which equates to 720 Greek feet, 72 Greek/Hebrew reeds of 10.5 English feet or 10 Greek feet, etc.
There were two very effective navigational systems encoded into the dimensions of the Great Pyramid that made positional plotting and dead reckoning across the featureless oceans of the world quite easy. These were based upon miles of 5250' & 5280' respectively.
The main reason why these "miles" were used was because of the way linear distances, in sea-legs, could be converted to circles using slight variations of PI. This meant that the navigator, using these miles or their league increases could produce whole number circumferences divisible by 360-degrees, when plotting distances covered, onto the scaled chart or slate. From the 360-degree scaled circle generated one could always keep track of the angle back to the point of departure or onwards to the destination, despite the zigzag course required when tacking on the wind, back and forth over a leyline to the destination.
Because most voyages were within the Mediterranean or Atlantic, the 152-mile shortfall, in assigning the equatorial size of the Earth to 24750-miles (of 5280' or 130680000'), or the 160 & 2/11ths-miles shortfall in using the 5250' mile (130636800'), were inconsequential. Very high degrees of navigational accuracy could be achieved by either system.
However, the ancient scientists still needed to have knowledge of the equatorial circumference (in factorable numbers) that drew very close to the actual size of the Earth (24902-miles), so used 12 X 12 X 12 X 12 X 1.2 miles of 5280' (24883.2-miles or 131383296'). This got them to within 18.8-miles of the actual equatorial circumference.
This mathematical progression works to breakdown the 131383296 number in much the same way. Therefore: 5280' X 12 = 63360' X 12 = 760320' X 12 = 9123840 X 12 = 109486080 X 1.2 = 131383296'
THE CODE 90-DEGREES OPPOSED.
Brian Campbell's second measurement of the dodecahedron hole produced the value of .88" and this relates to a second reading of the Great Pyramid's base length. If that length was read to be 756.25' (only 3" greater), then the navigational system that used the mile of 5280 English feet would come into play.
On the original, pristine and unblemished pyramid, all that would be required would be to mark the paving at the base of the pyramid to indicate these 3 additional inches of length. By this means, two circumnavigations of the Great Pyramid would equate to 6050' for 1-minute of Earth equatorial arc, 363000' for 1-degree of arc and 130680000' to describe the full equatorial circumference at 24750 English miles. This was the very effective "11" system of world navigation.
Using the formula of dividing the 756.25' length (9075") by 440, an Egyptian Royal cubit of 20.625" inches is produced (20 & 5/8ths)
William Flinders Petrie identified that an Egyptian Royal cubit, measuring, essentially, 20.625" (20 & 5/8ths) had existed. This length, read as miles of 5280' X 1200 = 24750-miles.
The reason why these two navigational methods were chosen was determined by the way linear distances converted to circles using slight variations of PI.
For the Greek mile method of plotting linear distances at sea, the PI formula was 22/7ths or 3.142857. Under this method, a Greek mile of travel (leg at sea) converted to a circle of 16500' (1-English league or 550" per degree of arc). Using a scale rule and compass over the map- chart, one could do excellent positional plotting and dead reckoning at sea by this method.
For the English mile method the rendition of PI used was 1728/550ths or 3.14181818. If traveling linear distances at sea in leagues of 16500', the circle produced was 51840'* or 144' per degree of arc. Again, this made positional plotting and dead reckoning quite easy, as a ship's navigator could keep track of the angle back to the point of departure or onwards to the destination accurately, despite many zigzag course changes over a leyline when tacking on the wind.
*Note: The slope angle of the Great Pyramid is 51.84-degrees, the base area that the Great Pyramid occupies is 5184 square Greek/Hebrew reeds. Within the duration of the Precession of the Equinoxes spanning 25920-years, the sum of 5184-years is 1/5th of the cycle.
* Note: Sir William Flinders Petrie measured the coffer in the Great Chamber of the Khafre Pyramid and accurately determined it had an outer length of 103.68-inches. This value doubled = 207.36-inches (207 & 9/25ths). In other words, the coffer was 5 cubits long using a cubit of 20.736-inches or 1.728-feet. The same cubit was represented in the dimensions of paving slabs at the base of the Great Pyramid. One of several codes carried in the length value of the coffer was the speed at which the Earth rotates in miles per hour. Therefore: 24883.2-miles ÷ 24-hours = 1036.8 MPH. Interestingly enough, the "Stirling Jug" of Scotland had a capacity of 103.68 cubic inches and this paralleled the Jerusalem Liquid Standard of the ancient Hebrews wherein a "Cab" was 103.68-cubic inches.
Also 20.736" read as miles of 5280' X 1200 = 24883.2-miles. This reading is only 18.8-miles short of the accepted circumference of the Earth that we use today.
In summary , the testimony of Julius Caesar that the druids lectured on, "the magnitude of the Earth and its divisions" is well-supported by this face of Brian Campbell's dodecahedron artefact.

A thin red, slightly elliptical, circle line is seen circumnavigating the rim edge of the centre hole, which was scaled to Brian Campbell's Vernier calliper dimensions in AutoCAD. It can be readily seen that the East-West diameter of .875"(7/8ths) and the North-South diameter of .88" (22/25ths) fit the hole edge with excellent accuracy.