
In November of 1897 a Monsieur
Roux made a remarkable archaeological discovery in a field north of Coligny,
Ain, France. He came across a buried statue of the Roman deity, Mars and with
it the badly broken up remains of what had once been a large bronze plaque.
There were in the vicinity of 153 individual fragments associated with the plaque,
most of which bore some form of writing, accompanied by calibration marks and
numerical values.
The writing itself, although inscribed by use of Roman letters and numbers,
was in an early language of the Gaul's and vaguely recognizable as terminology
used by the Druids to indicate moon phases or fests.
The Coligny Calendar is thought to have first been fabricated in about 50 A.D.
or about 100-years after the Romans made their unwelcome incursion into the
affairs of Gaul under Julius Caesar.
The utter fragmentation of, what should have been a timeless, durable plaque of bronze, suggests that it suffered intense violence at the hands of individuals who held it in tremendous contempt…possibly Romans wishing to enforce the Julian Calendar system…or more likely latter Roman Christians, wishing to enforce the edicts of the Church to obliterate all things pagan…including images of non Christian deities.
That any of these precious
metal parts survived at all is a miracle and one is given to speculate whether
or not some old Druidic priest gathered up the smashed remains of a cherished
scientific calculator then deposited them for safe keeping in the forest.
An age marked by scientific accomplishment was being eclipsed by a long, dangerous
age, where ignorance and brutality would muffle the wisdom of the old sages
and extinguish the accrued scientific knowledge of millenniums. The fragmented
Calendar of Coligny was destined to sleep undisturbed in its hiding place for
many centuries until a time when it could emerge into world that would wish
to preserve it.
So, what can the Coligny Calendar tell us about the pre-Christian astronomical/ mathematical endeavours of ancient, Druidic France?

Figure 1: There remains only 45% of the original bronze plaque, which makes
it difficult to decipher. The official measurements of the plaque are 1.48
metres long X .90 metres high (see: Astronomische Gesellschaft Meeting
Abstracts, Abstracts of Contributed Talks and Posters presented at the
Annual Scientific Meeting of the Astronomische Gesellschaft at Heidelberg,
September 14--19, 1998, talk #J12 by Harald Gropp).
A bronze frame surrounds the more centrally inscribed region, creating both outer and inner rectangles. The inscribed region of the plaque was divided up into 16 equidistant vertical columns and 8 box segments within each of those columns. This provided a total of 128 boxes in which writing, numbers, day-marks or symbols of notation occurred. A space beneath the top perimeter frame contained no inscribing throughout the top horizontal length of the plaque to a depth of almost 6 cm.
.jpg)
Figure 2: It's quite obvious that the Coligny Calendar was set out as an
accurate grid before any inscribing occurred. In theory there should have been
1830 of these small peg holes across the calendar. A total of 1770 holes would
have indicated phases of the moon through 60 lunar months (1770-days = 354 X
5...the lunar year is 354.375-days).
There were also two special "CIALLOS" regions, used for inserting intercalary days as needed. Although little is left of one CIALLOS region, we are still able to see that each represented 30-days (MAT). The fully titled months of the calendar plaque were, split into biweekly sections. Six of the months were composed of a total of 30-days. The other six months were composed of 15-days in the first biweekly section and 14-days in their seconf biweekly section.The double spaced CIALLOS regions (occupying space generally allotted to four months) number up to XV or 15-days, with another 15-days allotted to their ATENOVX appendages. This researcher considers that the CIALLOS regions were used in a wide variety of ways. This included one function designed to indicate 1/8th of a year (45.75-days) in a circular count within a CIALLOS that ended on the first day of MID SAM (46th day).
When the bronze alloy plaque was subjected to brutal forces of destruction, it had a natural tendency to fracture on the closely spaced holes (like a postage stamp). The following was the generalized, overall result.

Figure 3: Fragmentation of the ancient Druidic calendar occurred primarily
on the holes within the column lines. The intended dimensions of each column,
between monthly and bimonthly headings, can be accurately assessed to find the
measurement increment of the designers and, thereby, the overall measurement
standard that they used in their ancient society. It appears obvious that the
holes were used for inserting pegs, which indicated the daily phase of the moon
and position arrived at within the Sabbatical cycle. By this method, the positions
of upcoming events or fests could be marked out well ahead of time.
THE MEASUREMENT STANDARD.
European/ Mediterranean people have maintained a tradition of using the Sumerian/ Babylonian sexagesimal system of time keeping, with 24 hours in a day, breaking down into hours of 60-minutes, with minutes of 60 seconds duration. The system is made up of periods of 7-days for a week and an intermix of sexagesimal and septimal values.
The degree angle system that European/ Mediterranean people have traditionally used is, again, taken from the Sumerians and Babylonians. The value of 360-degrees in a circle, broken down into 60 minutes of arc, which are further broken into 60-seconds of arc has long been used…which raises an awkward question… whatever happened to the Sumerian/ Babylonian measurement standard and why was that not adopted by European/ Mediterranean peoples…?
A linear measurement standard must precede either time or degree-angle systems, as it's the most basic or rudimentary stage of any such 3-4 part package, which also includes weights and volume measures.
The fact is that the
"so-called" British standard of measurement
is also the Sumerian/ Babylonian standard and it was this same standard
that built the Pyramids of the Giza Plateau, the Octagon of Newark Ohio, Stonehenge,
as well as the geometric layout of Rennes Le Chateau in Southern France.
For European peoples, the measurement standard seems to have originated in Egypt
and to have spread from that cultural centre world-wide in antiquity…as
far afield as New Zealand at the base of the South Pacific. The Calendar of
Coligny was built to that Egyptian/ Sumerian/ Babylonian/ British standard and
contains coded values, which cannot be extracted or identified by any other
system of measurement.
The pre-dynastic mummies of Egypt show us that the people occupying the region at that early epoch were of European physiology, with brown, blond or red hair. The busts of the early Pharaohs of Egypt are, again, European in physical makeup or appearance and wall art of the tombs often depicts the people as blue or green eyed, of light reddish complexion, with red, blond or other European hair hues. Pharaoh Ramassees II, whose mummy is on display in the Egyptian Museum at Cairo, has reddish blond hair, as do many other Pharaonic Dynasty mummies from about 1500 to 3000B.C. or earlier. The Nubians finally overwhelmed Egypt in the millennium before the Christian era. Many former residents of Egypt, as well as their cousin peoples occupying satellite nations like Israel, had migrated to Europe as early as 5000 B.C., taking all of their sciences or weights, measures and volumes standards with them. Harps, bagpipes, plinn rhythm's, cultural symbolism, language, gods and many other expressions of their early society were carried with them along the waymark trails of North Africa to the Pillars of Hercules. The migrants crossed the Mediterranean at Gibraltar, then became the people of Europe. The oldest books and legends of counties like Ireland speak of their Mediterranean origins. The lengths and angles built into the Pyramids of the Giza Plateau, which edifices had formerly acted in the capacity of a Bureau of Standards, were immediately encoded into the landscapes of Europe. Hence, the 3300 stone markers of Carnac in Brittany, acting as a giant library of scientific codes, formerly used in Egypt, or the landscape marking in and around Avebury Henge, an appendage or related structure of which is Stonehenge
Let's look at the inbuilt coded values in the official dimensions of the Coligny Calendar:

Figure 4: The importance of these measurements on the outer or inner perimeters of the frame cannot be overstated.
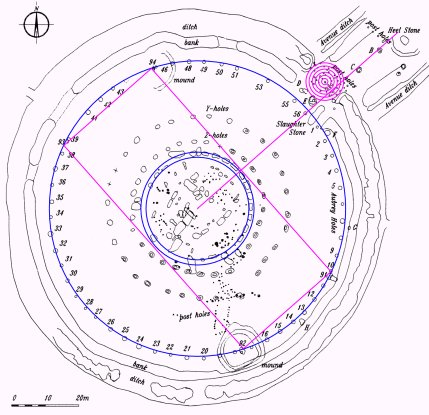
Figure 5: The Station Stones rectangle at Stonehenge was 264 feet long and 113.4 feet wide. It perfectly enclosed the Sarsen Circle in its width from 45-degrees azimuth to 225-degrees azimuth (the Sarsen Circle was made slightly elliptical to accentuate the 113.4-day lunar code…6804-days =113.4 X 60). Ninety degrees opposed to the 264 feet long eastern side of the Station Stones rectangle the sun rose on the day of the summer solstice…with "first glint" to the north of the Heel Stone at 48.6- degrees. Remember, the Coligny Calendar plaque is 4.86-feet in its outer edge lengths.
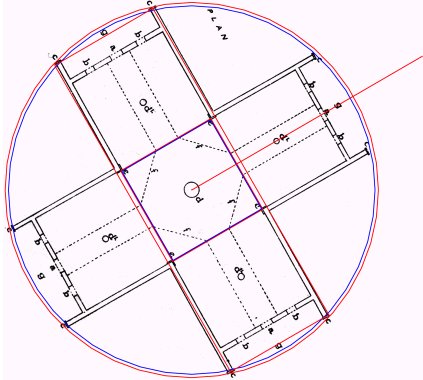
Figure 6: A strange former Maori meeting house, which had a pedigree back
to the mysterious Patu-paiarehe people … a civilization vanquished by the
Polynesian Maori. Many pre-Maori people of New Zealand fitted the description
of Europeans and the ancient mathematics found within the coded structures of
New Zealand attest to a strong cultural link to Europe and the Mediterranean.
The Crosshouse of Miringa Te Kakara seems to follow the identical surveying
methodology of Stonehenge in its astronomical coding.
The red rectangle, which runs to the outside of two wings, is 52.8 feet long
(26.4 feet X 2). The width of the wings (17.01 feet) is a strong lunar code
that helps identify the duration of the lunar nutation cycle (6804-days = 1701-days
X 4).
THE AGE-OLD METHOD BY WHICH THE CALENDAR OF COLIGNY WORKED.
The Calendar of Coligny was lunisolar, which meant that it took into account the cycles of the sun and moon simultaneously. It was designed to have these two utterly different duration cycles meld together at specific windows of time and stay abreast of both on a daily basis. The only way that the two cycles could be combined within a calendar system was to incorporate them both into a calendar spanning seven years.
Various theories and speculations have been raised about the need for adding intercalary months or making other complex adjustments in order for the Coligny Calendar to have some semblance of function. As it turns out there was nothing complex about the system and it could run indefinitely without any need for serious adjustment or intercalation. All that was required to keep the system accurate was the return of the full Moon every 29.53125-days (29 & 17/32nds), as well as seasonal observances of the solar equinoxes or solstices.
The system used by the Druids when the Calendar of Coligny was fabricated had been handed down to them for several thousand years and was based upon the age-old Sabbatical Calendar. This accurate, tried and true calendar system had been used in former homelands beyond the Eastern Mediterranean and had been carried from Egypt in about 5000-4000 BC when that land had been abandoned due to creeping aridity.
MEASURING THE MOON WITH A REED.
The oldest books or records contain clues about measurement standards in use during remote epochs. One such standard, the reed, was based, equally, upon the numbers six and seven used together. This means that any values derived could be divided equally by the numbers six or seven. The ancient measurement referred to was six Royal cubits (a cubit and a handbreadth) in length. We know from comparative analysis that the Hebrew (Royal) cubit referred to was 21-inches in length and that six such cubits formed a reed measurement of 10.5 feet or 126-inches. Mention to this very important, ancient reed measurement, semi-hidden within religious symbolism, is found in both the old and new testaments of the Bible. Here are some references to the reed measurement, which was still in use or known amongst the few remnant survivor Hebrews, following Israel's conquest by the Babylonians.
'and in the man's hand a measuring reed of six cubits long by the cubit and an hand breadth (see Ezekial 40:5).
1 And there was given
me a reed like unto a rod: and the angel stood, saying, Rise, and measure the
temple of God, and the altar, and them that worship therein.
2 But the court which is without the temple leave out, and measure it not; for
it is given unto the Gentiles: and the holy city shall they tread under foot
forty and two months. [The reed measurement
was 126-inches or 42" X 3 and was based
upon 6 X 7 = 42]
3 And I will give power unto my two witnesses, and they shall prophesy a
thousand two hundred and threescore days, [1260]
clothed in sackcloth (see Revelations 11: 1-3).
The Great Pyramid, which was built to be 756 feet in length, is 72 reeds long per side or 288 for a full perimeter circuit. The Khafre Pyramid, which was intended to have a design length 15/16ths the length of of the Great Pyramid, is 67.5 reeds per side or 270 for a full perimeter circuit. One of the most important functions of the reed measurement was to keep track of both the sun and moon during the Sabbatical Calendar cycle (2556.75-days...7 solar years...working alongside...2551.5-days...7.2 lunar years) or the Lunar Nutation cycle (6804-days). Before we can understand how the Druids kept track of both the sun and moon within the Calendar of Coligny counting system, we have to become fully conversant with the ages-old reed measurement and its attributes. It is the opinion of this researcher that the rods or staffs carried by the majority of astronomer Druids would have been half a reed in length (5.25 feet or 63 inches...one tenth of a Greek stadia).
With nothing more than a half reed rod in hand, suitably marked into finite incremental values, accurate calendar calculations could be completed by an adept, isolated Druid, in the same vein as a Druid with direct, daily access to the Calendar of Coligny brass plaque.
Here are some clues related to how the half reed (5.25 feet) can aid in all aspects of Sabbatical Calendar or Lunar Nutation cycle calculations:
SOLAR YEAR CALCULATION.
The Earth completes a circuit around the Sun every 365.25-days and this was the solar figure used in calendar calculations, although the ancient astronomers had refined the cycle to 365.2420-days.
It was considered needful that fluid, functioning and regulated society have a simple system of day counting, wherein there were always 7-days between Sabbaths. For this reason a "raw count" system was devised wherein there were 28-days in each month and 13 months in each year, culminating in a year of 364-days.
Rather than make adjustments
on an annual basis, the system was left to run unimpeded for 7 full years, by
which time a known error of 8.75-days had crept into the equation. This small
accumulating error didn't matter, as farmers were aware of the annual shortfall
and could adjust planting accordingly by a day or two if they so desired. They
could also observe the equinoxes, which marked the beginning of two seasons
or the solstices, which marked the centre points of the two additional seasons.
The main thing was that, under the convenient sabbatical system, society was
regulated on a week-to-week basis and no-one had to do any mental arithmetic
to figure out what day it was…like we in modern society must constantly
do in order to know the calendar date… under the odd, inferior system foisted
upon us by Roman Christianity...'thirty days hath September, April, June
and November. All the rest have thirty one, except February, which hath twenty
eight days clear and twenty nine each leap year'... Jeez!!!
It is because the Celts/ Britons and earlier groups followed the Sabbatical Calendar system that there were 13-months in their solar calendars, each marked by different trees… or, in other cases, animals.
At the termination of the 7th or Sabbatical Year (2548-days…7 X 364) a festival would begin, which would endure for about 9-days (8.75). During this period the solar count would come back into synchronization and the next sabbatical cycle could begin anew with everything fully corrected (2556.75-days). This same system of counting would be repeated for 7 X 7-years until the end of the 49th year. The synchronization applied equally to the lunar count and, at the end of each Sabbatical year or before the commencement of the 50th (Jubilee) year, the lunisolar calendar was, without any need for radical adjustment, running absolutely precisely.
"Calendar correction" festivals at the 7th year juncture might have been shorter in some regions of Europe or the Mediterranean, with a larger correction just prior to the 50th (Jubilee) year. The late era Hebrews appear to have observed one "double Sabbath" each year, which effectively added one day to the annual count, bringing it to 365-days per agrarian year. This would mean a correction at the termination of the Sabbatical Year (7th year) of only 1.25-days.
LUNAR YEAR CYCLE.
The moon completes a full circuit of the Earth every 354.375-days (to use the exact number of the ancient Druids, Egyptian or others) and the lunar year is, thereby, shorter than the solar year by almost 11-days. This disparity in the two cycles provided a seemingly difficult problem to overcome for anyone wishing to devise a calendar that followed the sun and moon simultaneously.
How this was done is indicated, in part, by the culling of Mistletoe on the sixth day after the full Moon…
THE SIGNIFICANCE OF THE MISTLETOE AND GOLDEN SICKLE CEREMONY.
Pliny the Elder speaks of a ceremony observed by the Druids, "which is to them the beginning of months and years (Sexta luna, quae principia…). This was the Mistletoe culling ceremony on the 6th day after the full moon. It's very significant that the terminology Pliny the Elder used was, "months and years".
Ancient Druidic Priests
of Britain are known to have gone into the forest on the 6th day after the full
moon to seek out an oak tree, which was inflicted by the semi-parasitic plant,
Mistletoe. For this ceremony the priests dressed in white robes and took with
them into the forest, a golden sickle with which they would "cut back"
or "cull" sprigs of Mistletoe from the venerated Oak.
A cloak would be laid out on the ground at the base of the tree, upon which
the cut sprigs of Mistletoe could fall…for the requirement was that they
were never to touch the ground. As the sprigs fell, other priests would dance
around the tree trunk singing, 'Hey derry down, down, down Derry!' which
literally signified, 'In a circle move we round the oak'.
Sadly, some of the most
misunderstood, misrepresented individuals in history are these brilliant astronomer
priests, for there was a profound astronomical/ mathematical reason behind this
otherwise "trite" ceremony.
The reason was borne out of a deep understanding of the solar & lunar cycles
throughout a period of 7 solar years and a need to synchronise the cycles in
order to have a functioning and perfected lunisolar calendar.
All of the symbolism in the ceremony points directly to the Sun & Moon and to a culling or cutting back of one to alleviate the burden of the other. Consider the following:
*Footnote: All of the special numbers encoded by lengths and angles on the Calendar of Coligny plaque or within the multitude of "open air universities" (standing stone complexes) were also incorporated into the "standards" of length, volume and weight within individual European nations of antiquity. Even within a nation itself, there could be many different expressions of the "mile" from province to province, but all were in a direct ratio relationship to each other. It seems obvious that, at some ancient era, each province was assigned (by Druidic priests?) the responsibility to preserve particular numerical values found within the overall parcel of scientific numbers. Some provinces would be preserving "lunar" information, whereas others would be preserving navigational values that were directly proportional to the equatorial size of the Earth. Here's an example of early Germanic lengths, based upon the Flemish system, that were in use in the 13th century AD and prior. The values given in metres are taken from "official" or expert literature and show only very small drift off the original, precise increments of more remote antiquity.
ALT KULMISCHE MASZ
1 Meile = 1800 Ruten ... 7777.24 Metres (this equals 25515-feet to a tolerance of 10.5-inches in 4.86 Greek miles of 5250-feet each. It is very obvious that this ancient Germanic/ Flemish/ Prussian system was fully founded on, what's come to be known as, the Greek-mile system). It was also mnemonically preserving lunar cyclic science.
1 Rute = 15 Schuh ... 4.3218 Metres (the Rute, which was in direct ratio to the Meile, was 14.175-feet. The Khafre Pyramid of Egypt, which was a "pyramid of the moon" accentuating the lunar cycle periods, was 2835-feet in perimeter value or 200 Ruten).
1 Schuh = 0.28812 Metre (The Schuh, of which 15 made up 1-Rute, was .945-feet or 11.34-inches). This was the increment of length that Greek Historian Herodotus was told about by Egyptian priests, who stated that the Great Pyramid was 800-feet long ... Therefore: 756-feet ÷ 800 = 11.34-inches. By using a rule of this length, the Great Pyramid base perimeter could give lunar cycle readings. Three side lengths of the Great Pyramid @ 756-feet per side = 2268-feet or 1134-feet X 2. The Great Pyramid is 3200-Schuh in perimeter and the Khafre Pyramid is 3000-Schuh.
1 Elle = 2 Schuh 0.57624 Metre (The Elle was 22.68-inches and there would be 1200-Elle in three base side lengths of the Great Pyramid). Alternatively, the width of the Station Stone's Rectangle at Stonehenge was 113.4-feet or 60 Elle).
THE DAY FOLLOWS THE NIGHT & THE WARM SEASONS FOLLOW THE COLD.
Julius Caesar observed that
under the Celtic system of time keeping:
"All the Gauls assert that they are descended from the god Dis, and
say that this tradition has been handed down by the Druids. For that reason
they compute the divisions of every season, not by the number of days, but of
nights; they keep birthdays and the beginnings of months and years in such an
order that the day follows the night."
Most writers versed in Celtic lore tend to accept that the Celtic new-year began in the colder months or on the first day of winter. Certainly this date was marked by significant celebration and became "All Souls Day" or Halloween. It does, however, seem impractical to do the mistletoe culling ceremony in the middle of a howling gale six days after the coldest day of the year. The only plausible time for clambering up oak trees, golden sickle in hand, or dancing around the oak's trunk singing as the mistletoe sprigs fell, would be at the height of summer. In consideration of what practical people are more likely to do, we can assume that the 12-day celebration was six days each side of the midsummer Solstice and not the midwinter Solstice.
The Calendar of Coligny bronze plaque commences its monthly titles with MID SAM...rendered elsewhere on the plaque as SAMON. This section is preceded by one of the two CIALLOS sections, which were separate, set aside, calculation boxes, not directly associated with the sequence of unravelling months. Although the very ancient forms of writing on the Coligny plaque are little understood and still the subject of much speculation, the term MID SAM, nonetheless, is identifiable as Mid Summer. Our linguistics scholars seem to be in very general agreement on that point:
'Samon - Ce mot est certainment en rapport avec le nom de l'été...Au sens large du terme, on peut interpréter samon par "estival", quoique, plus précisément, il désigne le "résumé", la recapitulation "de l'été.'
'Samon - This word is certainly in connection with the name of summer...In the broad sense of the term, one can interpret samon by "estival" [estival = summer *[adjective-adverb]], it designates the "resumption", the "recapitulation" of summer' (see: Les Druides du Québec © 2003 courriel : adboutios@hotmail.com ).
From an astronomical point of view it is essential that a (major, infrequent) calendar "fix" be an 8th of a year after the beginning point of a season, such that one can get an exact solar position time fix (Solstice). For further refining the precision of that fix, it should be made at the time when the moon (at rise and set) reaches its most extreme northerly or southerly positions on the horizon (each 18.613-years) at Lunar Major Standstill.
HOW THE CELTIC SABBATICAL SYSTEM WORKED PRACTICALLY IN AGRARIAN SOCIETY.
Romeo.
Lady, by yonder blessed moon I swear That tips with silver all these fruit-tree
tops,—
Julliet. O! swear not by the moon, the inconstant
moon, That monthly changes in her circled orb, Lest that thy love prove likewise
variable.
SO WHAT EXACTLY DO YOU WANT CALCULATE?
The Druidic system, as encoded into the Calendar of Coligny, offered a range of calculation options. These included:
WHY WAS THE CALENDAR OF COLIGNY SET OUT AS 128 BIWEEKLY SQUARES OR 64 MONTH SQUARES?
Each square represented a biweekly period of either 14 or 15 days, with some months accumulating into 29 days and alternate months into 30 days. Although this averaged 29.5 days per month, the priest astronomers knew it meant 29.53125-days in every case. It didn't matter that months were half a day over or under, as the returning full moon ironed out any discrepancies in the reading. So how do we know that the ancient lunar month was actually read as 29.53125-days? By assigning values of either 29.53125-days (lunar) or 30-days (solar), the progress of both the sun and moon could be monitored and plotted simultaneously.
These clues indicate that the Coligny Calendar is mathematically related to things happening on the Great Pyramid, as well as Khafre Pyramid…and that they are all encoding identical lunar methodologies. It also provides us with the essential formulas to make correct calculations over the Calendar of Coligny's central chessboard matrix, where full lunar months are set out in 64 squares, with special, multi-optional CIALLOS sections offering a range of differing calculation opportunities.

Figure 7: The columns are housed within a rectangle that is 4.536 feet long
and there are 16 columns. This means that each column is .2835 feet wide or
3.402 inches. The Khafre Pyramid was 2835 feet in base perimeter value or 34020
inches.
In the most fluid and straightforward usage of the Calendar of Coligny plaque (64-squares) it works perfectly within the confines of the 6804-day Lunar Nutation Cycle and a count of all squares will give a figure of 1890-days or one quarter of the duration of the Lunar Nutation Cycle.
THE SABBATICAL SOLAR CYCLE, USING AN INCREMENT OF 30-DAYS.
It seems apparent that the two *CIALLOS sections of the Calendar of Coligny were placed there to serve multiples of calculation functions and each could be pressed into service to represent two solar month of either 28 or 30-days or, even two lunar months of 29.53125-days if desired for demonstration purposes. By this means the bronze plaque, symbolically, offered blocks of 64 months per circuit in which to complete solar or lunar calculations manually. Such a provision would have been very valuable in teaching initiates the art of tracking the sun and moon periods within a lunisolar calendar system.
The sum of 63 solar months of 30-days duration = 1890-days or 270 weeks of 7-days each. This sum also represents 67.5 months of 28-days each (peasant or agrarian community months). To complete a full Sabbatical solar cycle (using months of thirty days) one would use 63 squares only and move through the plaque 1.35 times + 5.25 additional days.
To complete a 7.2 lunar year cycle (using months of 29.53125-days) one would use 64-squares and move through the plaque 1.35 times.
*Footnote: In a more literal or practical sense, one could not disrupt the natural flow of months by adding in intercalary months. These two CIALLOS sections of the calendar, obviously, offered separate calculating functions distinct from the unravelling, titled months. The best clue related to the primary function of the CIALLOS sections is found in the sub-title: SONNOCINGOS (the march of the sun).
Whereas the main body of the calendar plaque seems to relate numerically to the lunar month (29.53125-days), the two CIALLOS sections offer counts of 30-days each, more in keeping with one of two methods of doing the solar month count. The first CIALLOS section of the calendar is badly smashed and our scholars have tried to recompose the scant, remnant pieces of text by comparison with the 2nd CIALLOS section, which is more complete. This is how the two sections are now thought to have been in their heading titles:
DACAMONOS BUIS [First]
period of adjustment.
SONNOCINGOS [The march of the sun] [by addition of one]...Sonno [sun]...Cingos
[march].
MID XIII [thirteenth month complete] [carrying].
MATUS CCC LXXXV LATES [the duration of 385
days].
The title at the top of the second CIALLOS section is composed of five lines of text, as opposed to the first CIALLOS, which has only four. This one (with some reconstruction) says:
CIALLOS B[U]IS
[Second] period of adjustment.
SONNOCINGOS [The march of the sun].
AMMAN.M.M XIII [Duration of 13 months complete].
LAT CCC LXXXV [Day 385]
[AM]BANTARAN M [month between the two].
The second CIALLOS calculation or adjustment section occurring on the Coligny plaque after the 30th month. The term SONNO CINGOS means: passage or journey of the Sun, which would infer that the CIALLOS sections were set apart, primarily, to track the solar year. The 3rd line, AMMAN.M.M XIII is said to mean: 13-months completed. If (or whenever) this was read to mean solar months, then the inference under the Sabbatical count system was 13 months of 28 days = 364-days. If (or whenever) this was read to mean lunar months, then it equated to: 13 X 29.53125-days = 384-days (rounded very slightly to a full day). Under such circumstances of dual coding the solar and lunar aspects simultaneously for separate value readings, then the line that followed would simply mean 365-days for the solar count or 385-days for the day after the passage of 13-lunar months ... either CCC DOUBLE XXX + V = 365... or ... CCC LXXXV = 385.
It is easy to see why the ancient astronomers would wish to include this mnemonic reference to 384-days (under the guise of the day before 385-days), as it relates, in a dynamic coded sense, to the duration of the lunar nutation cycle of 6804-days. Therefore, 6804 ÷ 384 = 17.71875. This number doubled = 35.4375. As we know there are 354.375-days in a lunar year or twelve months of 29.53125-days. There are 19.2 lunar years in the 6804-day lunar nutation cycle. Alternatively, the lunar aspect of the Sabbatical calendar (7.2 lunar years or 2551.5-days) X 2 & 2/3rds = 6804. By using simple mathematical tricks or memory triggers at intervals within items like the Calendar of Coligny, the ancient scientists could always remember their parcel of astronomical and navigational numbers.
Tracking the solar cycle was considerably simpler than staying abreast of the more complex waxing and waning moon, oscillating to extreme positions on the horizon during an 18.613-year cycle. The CIALLOS sections seem to have been set aside primarily for solar counts or to serve a range of secondary calculation functions.
THE SABBATICAL SOLAR CYCLE, USING AN INCREMENT OF 30.4375-DAYS.
The solar month, when based upon one twelfth of the true solar year of 365.25-days = 30.4375-days. A count on the bronze plaque of 84 such (assigned duration) months would complete a solar Sabbatical cycle of 2556.75-days (365.25-days X 7). The sum of 62 such months would equate to 1887.125-days or 2.875-days (69 hours) less than 1890-days (189-days is 1/36th of the Lunar Nutation Cycle of 6804-days).
THE SABBATICAL SOLAR CYCLE, USING AN INCREMENT OF 30-DAYS.
If one considered that each named month had a value of 30-days, then a count of 63 such months would equate to 1890-days. Alternatively, 85.225 such months (85 months and 6.75 days) would equate to seven solar years of 365.25-days each and 85.05 such months (85 months and 1.5-days) would equate to 7.2-lunar years.
THE LUNAR SABBATICAL CYCLE, USING AN INCREMENT OF 29.53125-DAYS.
One should not be scared of numbers with long tails of decimals, such as 29.53125, as these are simple fractions that were easily within the capacity of the ancient mathematicians to express and work with. This number is, simply, 29 & 17/32nds. By assigning each of the titled month blocks (CIALLOS sections not included) on the bronze plaque a value of 29.53125-days (one lunar month to an accuracy of about 57-seconds), then 64-squares would complete 1890-days. Alternatively the passage of 86.4 such months would equate to the 2551.5-day count for 7.2 lunar years. An additional 5.25-days would equal 2556.75-days, the duration of 7-solar years.
THE SEVEN DAY WEEK, TWENTY-EIGHT DAY MONTH, AGRARIAN COMMUNITY COUNT.
For simplicity, the peasant communities worked to a 13-month year, with each month enduring for 28-days. If each block of the bronze plaque were considered to mark off 28-days, then 67.5 such months would equate to 1890-days and a count of 91.3125 such months (91 months and 8.75-days) would complete seven solar years. Alternatively, 91.125 such months (91 months and 3.5-days) would equate to 7.2-lunar years.
Except for teaching initiates how the solar aspect would work within monthly categories across the 60 titled months appearing on the plaque, it seems apparent that the solar cycle was mostly monitored within the two CIALLOS sections. These were composed of 30-days each, but offered the added option of using only 28 of the numbered counting holes if following the 28-day month X 13-months per annum, Sabbatical Calendar system.
Because the overall Calendar plaque is made up, equally, of MAT months (30-days) and AMN months (29-days) averaging 29.5 days. The option was certainly available to, simply, consider all titled months to be only solar months of 30-days each, if that was found to be desirable for a calculation option. In another instance one could consider that all titled months were only lunar @ 29.53125-days each, or then immediately switch to representing each titled month as only 28-days duration each under the Sabbatical system of solar month counting. The plaque was set out in a very versatile manner and could act as a calculating matrix for any one of several things simultaneously, including acting as a static calculator of solar year and lunar year positions arrived at within the lunar nutation cycle.
THE 18.613-YEAR (6804- SOLAR DAY) LUNAR NUTATION CYCLE…MAJOR STANDSTILL TO MAJOR STANDSTILL.
During a period of 18.613-years the moon moves from major standstill (most northerly and southerly rising and setting positions) to minor standstill (least northerly and southerly rising and setting positions) then back again to major standstill. Visually following this wide span to short span oscillation of the moon, up and down the horizon, was of tremendous importance to ancient astronomers, who were using the daily position of the moon (the way the moon was crossing the Earth's path and its exact position…declination) to make long-term weather predictions.
The moon, because of its
intense gravitational tug on our atmosphere, is the largest single contributor
to changing weather patterns across the face of the globe and can be used with
excellent relative accuracy to foresee upcoming weather fluctuations.
This infers that astronomer/ priests of the old orders could foretell when periods
of drought, flooding, intense storms, early and late frosts, etc., were most
likely to occur. Practical preplanning, based upon regional experience, could
limit potentially drastic effects when the moon's position was portend to coming
danger.
Farmers could be forewarned to plant earlier or later…or to leave the land
lying fallow through a dangerous season. The loss of seed stocks or crops could
be minimized and the chances for successful outcomes in agrarian communities
optimised by knowledge of sound scientific principles of lunar cause and effect.
On all standing stone circles that this researcher has studied, the categories of navigation and the cycles of the moon seem to be more greatly accentuated and important than the solar observations.
The Calendar of Coligny, offers a total of 60 titled month in one traversal of the plaque. In a symbolic sense it offers 64 months if one counts the space occupied by the two CIALLOS sections. However, the sequence of months, unravelling in chronological order, show that the plaque was built to serve the dictates of the 6804-day lunar nutation cycle. The sum total of 64 months (one full traversal of the plaque, plus four additional months of 29.53125-days) is 1890-days and 3.84 full panel counts of months (230.4 months...230 full months plus 12-days) resolves to 6804-days. By following this cycle on a daily basis, the phase of the moon and its exact changing position, in relation to the Earth's plane (declination) could always be known or predicted exactly by mathematical calculation (even years ahead of time).
Again, the degree of accuracy
between the use of 6804-days to describe the lunar nutation cycle and that of
the precise cycle at 6798.36-days amounts to very close to the 5.25-day increment…
6804 - 6798.36 = 5.64-days.
The standard increment of 5.25-days, used to adjust either the sun or moon values
throughout (and at the end of) the lunisolar sabbatical cycle, can again be
pressed into service. The reed measurement increment (10.5 feet) appears to
have been devised specifically to address the needs of solar and lunar calendar
calculations
It's interesting to note that the Reed measurement worked just as effectively for the ancient civilisations in their maritime navigation, based upon a 6&7 reading of the Earth's equatorial circumference (i.e. 12 X 12 X 12 X 12 X 1.2 Greek miles of 5250 feet each, which is either 24882.2 Greek miles or 24741.81818 British standard miles of 5280 feet each). This reading, which is the same system encoded into the perimeter dimensions of the Great Pyramid (756 per side...3024 feet for 4 sides...1/2 a minute of arc in the Earth's equatorial size) is fully divisible by the reed measurement of 10.5 feet.
THE 19-YEAR METONIC CYCLE OF THE MOON.
There is a wonderful conjunction between the sun & moon cycles at the resolving point of 19 solar years or 235 lunar months, which is referred to as the metonic cycle of the moon. This could have been used as a fine-tuning and adjustment cycle to maintain accuracy within the Coligny Calendar.
THE LAYOUT OF LUNAR MONTHS…AND MARKERS INDICATING SIGNIFICANT MILESTONES.
The Calendar of Coligny
is in a dismal condition with well over 50% missing. Despite this inconvenience,
sufficient remains for researchers to identify the assigned names for each lunar
month and whether or not they were designated as 29-day months (ANM)
or 30-day months (MAT). The day-count assigned to each month is clearly
seen by the numbered "holes" running vertically down the side of each
column box. All numbers are in Roman numerals, ranging from I, II, III, IIII,
V, VI, VII, VIII, VIIII, X, XI, XII, XIII, XIIII, XV.
The boxes are read from the top left corner downward and from left to right
across the bronze plaque.
The 12 names or titles for lunar months on the calendar are:
SAMON (MAT), DVMAN
(ANM), RIVROS (MAT), ANAGAN (ANM), OGRONN (MAT),
CVTIOS (MAT), GIAMONI (AMN), SIMIVIS (MAT), EQVOS (AMN),
ELEMIV (AMN) EDRINI (MAT), CANTLOS (AMN) = 354 days (the lunar
year is 354.375-days).
Each of the above titles is preceded by the letter M.
The first SAMON month has a special designation, MID SAM.
Each of these months is split into two parts with the word ATENOVX inserted between the biweekly periods. The word ATENOVX would have appeared on the calendar 62 times.
The first box of the calendar contained the title, CIALLOS, which is described in most articles about the perceived workings of the calendar as an intercalary month of 30-days (MAT). These titles appear at the beginning of the calendar and also at the end of the 30th month. A CIALLOS occupies the same space as would normally be alloted to two months. Each half of a CIALLOS numbers to XV or 15-days total, including its ATENOVX appendage, also numbering 15-days. It appears likely that these regions were set aside for a variety of special calculation functions, but were mainly for monitoring the solar years.
The term TRINoX SAMO sindiv seems to refer to the 3-nights per month when the moon does not appear… the 3 nights of darkness on the 14th, 15th, & 16th days after the full moon.
Other terms in various boxes
include, PRIN LOVDIN, AMB, IVOS, AMB IVOS, INIS R, DVMAN exo IVOS.
Special symbols like ƒJJ or JƒJ or JJƒ sit
adjacent to particular lunar markers and possibly refer to lunar declination.
SOME MISCONCEPTIONS ABOUT INTERCALARY MONTHS.
The Calendar of Coligny is in a very fragmented state, with many column titles completely missing. Despite this imposition, researchers have been able to set out the titles in order and assign each of the months their correct 29 (AMN) or 30 (MAT) classifications.
Some prominent researchers have concluded that the 2 CIALLOS sections represent 2 intercalary months with a value of 30-days each. From this they conclude that the ancient Druids were working a system of 62 lunar months duration, which equated very closely to 5 solar years. This, they say, could also be broken down into two segments within the calendar of 2.5-years duration each.
The major problem with this theory is that the ancient people could not disrupt the chronological unfolding of their year, as a named month was locked to a specific season, following on the heels of another named month, equally locked to its season. Even today we can't disrupt that flow and any officials who came along and told us that they were going to insert a CIALLOS month between October and November would quickly get the bum's rush. If such drastic intercalation were implemented, the titled name describing mid summer would, eventually, make no sense at all. It would, eventually, describe midwinter.
Intercalation of time has
to be limited to a few days of adjustment here and there at the end of a long
period (like seven full solar years). The system has to be so well designed
and closely accurate that any intercalation, to bring the calendar systems in
line with the true duration of the solar & lunar cycles, is only very minor.
Benchmark points, for adjusting any drift or slippage in accuracy are provided
by the solar equinoxes and solstices. By observing them regularly, precision
in the calendar count is assured.
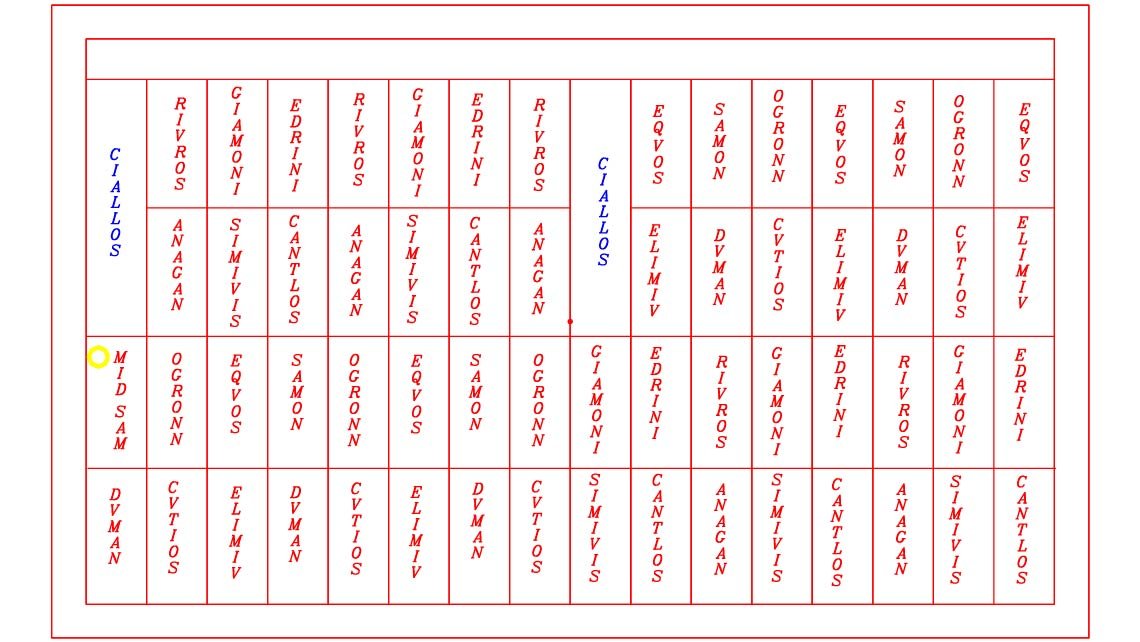
Figure 8: A reconstruction of the Coligny Calendar, showing the placement
of all the months and including the "CIALLOS" regions. The
yellow orb marker is this researchers way
of indicating the ceremony of cutting mistletoe on the 6th day after the full
moon.
A very visible and deliberately made dot indentation mark, sitting to the right of the 6th day of MID SAM, might indicate the mistletoe ceremony, which was designed to accumulate 5.25 days (6-days) such that sun & moon periods would resolve together to a 7 solar year count. This prominent dot indentation marker would also indicate the end day of (what became) the Christian 12-days of Christmas period (stolen off the pre-Christian Pagans). From this day (six days after the summer solstice) 7.2 lunar years would terminate on the same day as 7-solar years running from the summer solstice.
These dot indentation markers sit to the side of VI in MID SAM, as well as to the side of VIIII in the CIALLOS square preceding. The dot indentation for 6-days before MID SAM is seen slightly above and to the left of VIIII (9th day of the CIALLOS / ATENOVX or 6-days before MID SAM). There are many more of these dot markers across the length and breadth of the plaque and situated adjacent to specific numbers in the day count. They all appear to represent purpose placed positions or milestones arrived at. (High resolution photo supplied by Rudi Diesvelt).
From
this dot indentation evidence it appears very likely that the 12-day observance,
situated each side of the summer solstice and culminating in the mistletoe culling
ceremony, was positively marked on the Coligny plaque.
SUMMARY OF OPTIONS.
Each of the options listed allowed for highly accurate forecasts as to when significant dates would be achieved in terms of lunar, solar and calendar events. Everything was calculable in reasonably finite terms with no large lumps of residual error to juggle or discard in the final adjustments. Layers of interrelating cycles could be dealt with separately or in partnership, linked by mathematical ratios.
Certain durations, like the lunar nutation cycle, were allowed to carry a small amount of known error during their term such that convenient mathematical progressions, for lunisolar calculation purposes, were made available. At the end of cycles, however, the known, small degree of error was eliminated with utter ease, such that layers of cycles, tied to the lunar nutation cycle foundation, could recommence in perfected unity.
There was no need for the drastic intercalation of whole months to make the ancient calendar system function, as suggested by some researchers, only the occasional accumulations of 5.25-day offsets between Sun and Moon each 7 solar years. These convenient, purpose-made clumps of 5.25-days, were ultimately adjusted out during festivals. The greater determinant cycle was drawn back to its exact 6798.36-day duration, then relaunced, to accumulate a known error of only 57-seconds per lunar month, for the next Sabbatical period before adjustment.
The Sabbatical calendar method was fashioned to very convenient, near perfect numbers, which were used to describe lunisolar attributes in terms that functioning humankind could understand, in the day to day running of their affairs. Events in the heavens dictated the day and hour when the greater cycle ended and the new cycle commenced its flow, carrying no burden of calendar error in its wake.
THE DOT INDENTATION CODES (MEMORY TRIGGERS).
We'll make a start on these and add more as time permits. There are many of these dot markers across the length and breadth of the Calendar of Coligny artefact to analyse for the extraction of number codes. As long as the dot indentations are clearly identifiable, the codes that they carry in their positions are easily recognised.
Indented into specific positions, adjacent to or straddling particular numbers, the artisan astronomers who built the Calendar of Coligny have placed small dot markers. These indicate that significant milestones have been reached in the count of days and that, either, the moment arrived at within the calendar cycle is of great importance, or else the "number" exhibited is one of the special ones, much used in the general calculation endeavours of their ancient society. Let's look at the surviving dot indentations and identify the coded purposes of these positions, one by one:
1. The two dots relating to six days before the summer solstice and six days after have already been discussed and these seem to relate directly to the 12-days of observance, ending with the mistletoe ceremony.
2. MID SAM ATENOX @ X (day 10). This position codes the numbers 25 or 25.2. In the lunar nutation cycle of 6804-days, there are 27 periods of 252-days. The base length of the Great Pyramid is 756 feet (252 X 3). The sum of 252 feet is 1/24th of 1-minute of arc for the equatorial size of the Earth (12 X 12 X 12 X 12 X 1.2 Greek miles of 5250 feet). There are 2.5 X 252 feet in a Greek long Stadia of 630 feet, etc. In hours, the sum of 252-days = 6048 hours, which in feet represented two perimeter circumnavigations of the Great Pyramid's base or 1-minute of equatorial arc under the 6&7 family of numbers used in navigation. All of the surviving Greek distance measurements (based upon the reed of 10.5 feet) were part of the 6&7 number family.
3. DVMAN @ about XIII (day 13). This position seems to bear a marker and it would make sense if it did. The dot indentation seems to be indicating 42.525. This is a very important lunar progression and there would be 160 periods of 42.525-days in 6804-days or 60 such periods in 7.2 lunar years.
4. DVMAN ATENOX @ XII (day 12). The dot is quite faint and in shadow to the left of the number, but if it is there it would make perfect sense. This position would code 56.7 (18.9-days X 3), which is a dynamic lunar number. There would be 120 periods of 56.7-days in the lunar nutation cycle of 6804-days (360 periods of 18.9-days). Alternatively, there would be 45 periods of 56.7-days in the Sabbatical calendar lunar period of 2551.5-days (7.2 lunar years). These values (120 & 45) would indicate that the lunar periods could be easily tracked on a 360-degree compass, based upon 18.9-days per degree. The sum total of hours in 18.9-days is 453.6. The vertical, design height of the Great Pyramid from its base to the flat surface of its platform floor on top is 453.6 feet (it never had a capstone). The sum of 453.6 ÷ 22* = 20.61818182 (which in inches is the official length of an Egyptian Royal cubit). This value X 1200 = 24711.81818 (which, in British miles of 5280 feet, was the equatorial circumference of the world as encoded into the base dimensions of the Great Pyramid...where one circumnavigation is 1/2 of a minute of arc). The sum of 24711.81818 British miles = 24883.2 Greek miles of 5250 feet each (12 X 12 X 12 X 12 X 1.2 = 24883.2).
*Footnote: The flat floor atop the Great Pyramid measures 44' X 44' and 22' is half that side length value. A clever mnemonic code, using the "hour" value of 18.9-days, allowed the Druid readers of the Calendar of Coligny to remember the age-old formula leading to calculating the equatorial size of the Earth. Under the old system, lunar values will automatically dovetail into, and are synonymous with, the values used to describe the size of the Earth.
5. DVMAN ATENOX @ XIIII (day 14). This position positively codes 58.32. The sum of 58.32-inches was 5 Roman feet of 11.664 inches each. The measurement was once used universally by the cousin nations as a distance increment or in various calculations to do with the lunar cycle and navigation. There would be 116.66666 periods of 58.32-days in 6804-days or 43.75 such periods in 7.2 lunar years. The Roman foot could be quoted variously as 11.664 or 11.66666, depending on the type of calculation that was being done.
It can be estimated with reasonable confidence that in the first section of RIVROS three special numbers would have been recorded. These were: 61.8, 63 & 64. The ancient Druids would have needed to remember the reciprocal value of PHI (.6180339) in order to fashion all of their volume tubs (bushel barrels, etc.) for use in the market places. The formula for making the bases was 10-inches ÷ 1.6180339 (PHI) = 6.18034 inches. A correct bushel barrel was 3 X 6.18034 inches in diameter (18.54102) across its circular base (270 square inches of surface). This, in conjunction with sides that rose to 8-inches above the tub floor, gave a cubic capacity of 2160 cubic inches for a bushel of dry grain produce. The number 2160 is a multi-use code of antiquity...the sun spends 2160 years in each house of the zodiac during the precession of the equinoxes, etc.
There are 108 periods of 63-days in the lunar nutation cycle. There are 40.5 periods of 63-days in 7.2 lunar years (2551.5-days). There are 1512-hours in 63-days and 1512 feet represented the distance for two sides of the Great Pyramid (756-feet per side). In the calendar counting system, 63 solar months of 30-days = 1890-days and 3.6 such periods completes the lunar nutation cycle. Alternatively, 64 periods of 29.53125-days (lunar month) = 1890-days. If the side length of the Great Pyramid (756 feet) is divided by 64 then the result is 11.8125 feet. There are 118.125-days in 4 lunar months. The Khafre Pyramid (Egypt's Pyramid of the Moon) is 708.75 feet long. One quarter of its side length is 118.125 feet.
6. RIVROS @ XIIII (day 14). This position is probably coding 1/5th of a solar year or 73.05-days. There would be 35 periods of 73.05-days in 7-solar years. Alternatively, this position would have been used in mnemonic reference to 72.9-days. There would be 35 periods of 72.9-days in 7.2 lunar years. The sum of 7.29-inches was 1/8th of a Roman Pace of 58.32-inches.
7. RIVROS ATENOX @ I (day 1). There appears to be two dot marks here in close proximity and they probably mean 75 & 75.6-days respectively. The lunar nutation cycle duration ÷ 75 = 90.72. The length of the Great Pyramid in inches is 9072 (756 feet). Alternatively, there would be 90 periods of 75.6-days in 6804-days. There would be 34.02 periods of 75-days in 7.2 lunar years. The Lunar nutation cycle (6804-days) ÷ 2 = 3402-days. There would be 33.75 periods of 75.6-days in 7.2 lunar years (the lunar duration within the Sabbatical calendar system). The value 33.75 provides an important lunar and navigational progression.
8. ANAGAN...midway between V & VI (days 5 & 6). This position was, undoubtedly, dual coded to mean 93.33333-days and 94.5-days respectively. Both values provide important progressions that relate directly to the lunar period or to navigation. There would be 72 periods of 94.5-days in the lunar nutation cycle. There would be 27 periods of 94.5-days in 7.2 lunar years. There would be 72.9 periods of 93.33333-days in 6804-days. The Bush Barrow Lozenge artefact of Southern England is 7.29 inches long (1/8th of a Roman Pace)* and this value is a part of a strong lunar and navigational progression, which includes the values for the Roman foot (11.664-inches) and Pace (58.32 inches) measurements. The Roman Stadia was 7290-inches (125 Paces).
*Footnote: The Bush Barrow Lozenge and Clandon Barrow Lozenge artefacts pre-date Roman Britain by a couple of thousand years. This shows that the so-called "Roman" measurement system was already being used universally by the Neolithic Age cousin nations, long before there was any semblance of a Roman civilisation.
9. ANAGAN @ XII (day 12). This position is, without doubt, coding 1-second of equatorial arc (100.8 feet) for the Earth under the Great Pyramid's geodetic system. Alternatively, there would be 67.5 periods of 100.8-days in the lunar nutation cycle. There would be 25.3125 periods of 100.8-days in 7.2 lunar years. The value 25.3125 is a part of a very important lunar and navigational mathematical progression. The Great Pyramid's base perimeter measurement (3024 feet) was 100.8 feet X 30. Alternatively, there were other geodetic systems, based upon various number families. This position would also be used for mnemonic reference to 1-second of arc under the other systems. One of these was the "true" equatorial size of the Earth, which was 12 X 12 X 12 X 12 X 1.2 miles of 5280 feet. Under this reading, one second of arc is 101.376 feet, which is the width of the stybolite platform upon which the Parthenon of Athens sits.
10. ANAGAN ATENOX @ I (day 1). There should once have been a marker here to code 105, in homage to the reed measurement. The position is now so corroded and damaged that it is not possible to see if an indentation marker was placed at this point.
11. ANAGAN ATENOX @ VI (day 6). This codes the number 110, or a primary component in the "11" number family, which was at the basis of the ancient league (16500 feet...3.125-miles, the mile...5280 feet, the furlong...660 feet, the chain...66 feet, the rod or perch... 16.5 feet, the fathom...5.5 feet (original) and the link... 7.92 inches.
12. OGRON ...faint mark midway between XIII & XIIII (days 13 & 14). This is dual coding both 131.25 & 132 simultaneously. The value 131.25 is an offshoot of the very important "Reed" measurement code, which relates directly to the lunar periods or the equatorial size of the Earth. If 6804-days is divided by 131.25, then the total derived is 51.84. The slope angle of the Great Pyramid is 51.84-degrees. The azimuth angle upon which the huge Octagon earthworks complex of Newark, Ohio was built is 51.84-degrees. The sum of 51.84-years = 1/500th of the 25920-year duration of the Precession of the Equinoxes. The linear distance of one ancient British league (16500 feet) converts to 51840 feet using an ancient navigational rendition of PI @ 3.141818182. If the sum of 2551.5-days (7.2 lunar years is divided by 131.25, then the result is 19.44. This was a very important navigational value and the distance of 19.44-miles (Greek or British...depending on which number family navigation was being completed under) was 1/1280th of the equatorial size of the Earth. The value of 131.25 feet = 1/40th of a Greek mile of 5250 feet.
The value 132 relates directly to the mile of 5280 feet and 132 feet = 1/40th of a mile. The side distance across Stonehenge of the station stones' rectangle is 264 feet (132 X 2) for the long side of the rectangle.
13. CVTIOS ATENOX...an important double marker sits alongside IIII (day 4). This codes 166.66666, which value was much used in antiquity. In any 3, 4, 5 triangle the "Adjacent" will always be 1.666666 less than the "Hypotenuse" and the "Opposite" will be 1.25 less than the "Hypotenuse".
14. CVTIOS ATENOX @ XI (day 11)... This marked position is seen to be in the higher levels of of the XI segment, inferring a number greater than 172.6, but less than 173. The position codes one of the most useful numbers of antiquity, 172.8. This was much used in navigation. The Great Pyramid's base perimeter length was 3024 feet (1/2 a minute of arc). 1728 Hebrew or Celtic Royal cubits of 21-inches each = 3024 feet. The lunar Sabbatical calendar period of 2551.5-days ÷ 86.4 (1/2 of 172.8) = 29.53125-days (a lunar month). The 6804-day period ÷ 172.8 = 39.375-days (945-hours) and a root value of an important lunar progression. There are 1728 cubic inches in a cubic foot.
15. GIAMONI ATENOX...between IIII & V (days 4 & 5). This midway indentation code is for mnemonic reference to 196.875-days. There are 6.66666 lunar months in 196.875-days. There are 12.96 periods of 196.875-days in 2551.5-days (7.2-lunar years). Note: the value 12.96 is half of 2592. The Precession of the equinoxes was anciently calculated to endure for 25920-years (360 X 72). The duration of 6804 ÷ 196.875 = 34.56. The outer rim of the Sarsen Circle at Stonehenge (11.52 feet per lintel X 30 lintels) = 345.6 feet. The Greek Didrachma commercial weight was 196.875-grains. The Roman Uncia was two Greek Didrachma. The Romans also used the Egyptian Beqa (Roman Semuncia), for their gold standard, which was 196.875-grains. The Greek Tridracma was also 196.875-grains.
16. SIMIVIS @ XI (day 11). This is a very significant code that relates to the equatorial circumference of the Earth. The value coded into this position of the Calendar of Coligny was 217.728. Under the Great Pyramid's geodetic system, the Earth was said to be 12 X 12 X 12 X 12 X 1.2 Greek miles of 5250 feet each in circumference or 130636800 feet. The value of 217.728 feet is a 600,000th part of that length. The length of 217.728 feet would equate to 224 Roman feet of 11.664 inches each, for a "Roman" equatorial reading of 134400000 Roman feet. This value would also equate to 124416000 Greek feet of 12.6-inches each. A tradition that was handed down until mediaeval times stated that 75 Roman miles (4860 feet each) was 1-degree of equatorial arc under their navigational system. The value of 217.728 feet of 12-inches would equate to 207.36 Greek feet. One of 3 main Egyptian Royal cubits is considered to have been 20.736-inches or 1.728 feet.
Under (what much later became known as) the British league system, based upon the number 11, the Great Pyramid's base perimeter was considered to be 3025 feet (instead of 3024) for 1/2 of a minute of arc. This gave an equatorial circumference value of 24750 British miles of 5280 feet (11-series miles). Under such a reading, one 600,000 part would be 217.8 feet.
17. SIMIVIS ATENOX between VI & VII (days 6 & 7). The number coded into this position is 229.166666 (2750 ÷ 12). Under the Great Pyramid's league navigational system (3025 feet for 1/2 a minute of arc) the Earth was considered to be 24,750-miles in circumference. Under this system, 1-degree of arc was 68.75-miles, which is 22.9166666 miles X 3. Alternatively, under the Great Pyramid's Reed navigational system (3024 feet for 1/2 a minute of arc) the Earth was considered to have a circumference of 24741.81818- miles of 5280 feet and 1-degree of arc was 68.72727272-miles (362880 feet...note the perimeter value of the Great Pyramid @ 3024 feet is 36288 inches) The 68.72727272 value is also 74.666666 Roman miles. A third of 68.72727272-miles is 22.90909090-miles (115200 Greek feet or 23.04 Greek miles). Under the "true" equatorial reading (24883.2-miles of 5280 feet), one degree of arc was 69.12-miles. A third of this value was 23.04-miles. The reason behind having different geodetic systems was for ease of navigation, using a particular number family in preference to another. The Greeks and others seemed to prefer the 6&7 (Reed based) system, whereas the "11" (league & mile) system survived in Britain.
18. SIMIVIS ATENOX @ XV (day 15). This indentation marker sits at the end of 8 lunar months for a reading of 236.25-days, which is 5670 hours. There are 10.8 periods of 236.25-days in 2551.5-days and 28.8-periods of 236.25-days in 6804-days. The Khafre Pyramid of Egypt is 15/16ths the base length of the Great Pyramid and has a design perimeter of 2835-feet. The sum of 2835 ÷ 12 = 236.25, which is also 22.5 Reeds There would be 12.8 increments of 236.25 feet in the perimeter of the Great Pyramid.
19. EQVOS ATENOX...midway between XI & XII (days 11 & 12). This position is in homage to the very important lunar and navigational number 262.5. The sum of 262.5 feet = 25 reeds. There would be 9.72 periods of 262.5-days in 2551.5-days and 25.92 such periods in 6804-days. The Heel stone at Stonehenge sits 259.2 feet from site centre. There are 25920-years in the precession of the equinoxes, etc. The sum of 262.5-days = 6300 hours. The giant Atur measurement of Egypt was, undoubtedly, 5-Greek miles or 26250 British feet.
20. EDRINI ATENOX...midway between XIII & XIIII (days 13 & 14). This codes the very important navigational and lunar number 324. There are 21-periods of 324-days in 6804-days. There are 7.875 periods of 324-days in 2551.5-days.
21. CANTOS...between XI & XII (days 11 & 12). The number coded here is 336, which is a very important navigational value. The length of 33.6 feet is 1/3rd of 1-second of arc under the Great Pyramid's geodetic system (100.8 feet per second). There would be 9 increments of 336 feet in the 3024 feet perimeter of the Great Pyramid.
22. CANTLOS ATENOX @ XI (day 11). This position is coding 350-days since MID SAM. There are 7.29 periods of 350-days in 2551.5-days or 19.44 such periods in 6804-days. The increment of 7.29-inches is 1/8th of a Roman Pace and the length of the Bush Barrow Lozenge artefact. There would be 3 X 19.44-inches in a Roman Pace of 58.22-inches. There would be 12.8 increments of 1944-miles in the 24883.2-mile equatorial circumference of the Earth.
23. SAMON @ VIIII (day 9). This is coding 364-days or the end of a solar year under the Agrarian counting system of 13-months of 28-days duration each = 364-days.
24. SAMON...midway between X & XI (days 10 & 11). This appears to be a marker and, if so, would indicate 365.25-days or the true duration of a solar year.
25. DVMAN...midway between VIIII & X (days 9 & 10). This is coding a very important lunar progression value of 393.75. There would be 6.48 periods of 393.75-days in 2551.5-days or 17.28 such periods in 6804-days. A distance of 393.75 feet would equate to 4725-inches. Under the Khafre Pyramid's 3, 4, 5 triangle method of construction, half the base length was 354.375-feet (adjacent), the vertical height was 472.5 feet (opposite) and the face slope was 590.625 feet (hypotenuse). Under the Hebrew Jerusalem weight (light) standard, a Talent was 472500-grains. A Roman Libra was 4725 grains. Alternatively, a Greek Commercial Talent was 590625-grains. In more direct reference to the 393.75 value found on the Calendar of Coligny, a Roman Uncia was 393.75-grains. The sum of 393.75 British feet would be 375 Greek feet or 37.5 Hebrew Reeds. This would also be 229.1666666 Egyptian Royal Cubits of 20.61818182 inches (refer back to 17. SIMIVIS ATENOX in this list).
26. DVMAN ATENOX @ I (day 1). By this point 13 full months have been completed, plus the first half of DVMAN = 29.53125 X 13 + 15 + 1 = 400. This is therefore indicating the passage of 400 days in the calendar count. There would be 400 X 17.01-days in 6804-days. There would be 150 X 17.01-days in 2551.5-days. The sum of 17.01 British feet would equate to 17.5 Roman feet of 11.664-inches. There would be 16.2 Greek feet of 12.6-inches each in 17.01 British feet.
We have now completed 14-months of the 60 repeating months across one sweep of the calendar plaque. There, visually, seem to be other indentation markers strewn amidst the 14-months dealt with in this analysis, but they are obscure and don't rate further commentary until archaeologists at La Musée de la Civilisation Gallo-Romaine positively verify their existence. This analysis is based upon viewing a high resolution photo, but, even at that, some further, possible indentation markers in the area already analysed are not 100% apparent. There are, however many more that are positively there on the plaque, yet to consider, survey and extract the codes from, beyond these first 14-months.
© To be continued:
Martin Doutré.