THE TUTORIALS CONTAINED WITHIN THE OUTLYING MOUND POSITIONS.
Of the 120 or so mounds counted by early settlers, the former positions of only 104 are now known. In addition to the "lost" mounds of the Cahokia complex, there was another large batch on the banks of the Mississippi River in Old St. Louis, Missouri. Technically, these other 27 or so mounds were associated outliers or yet another expansive set of classrooms. The giant mound over in St. Louis was dubbed "Big Mound" and it related back, by way of a coded distance and angle, directly to the centre of Monk's Mound.
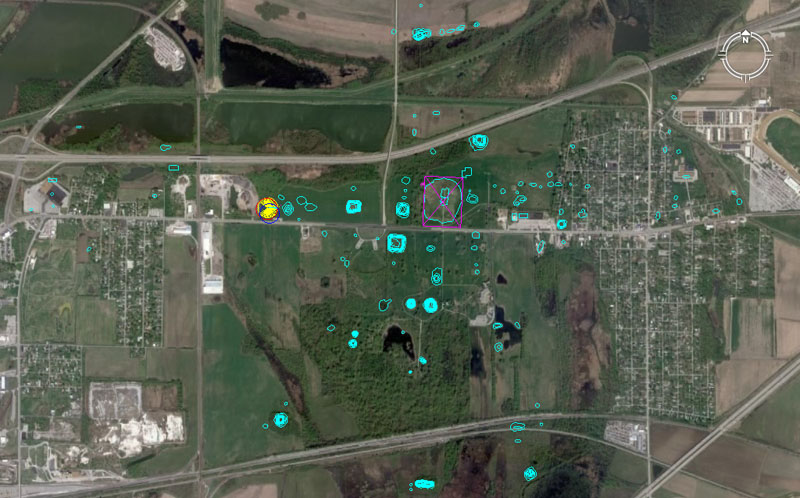
In this picture the Google Earth image of Collinsville, Illinois is overlain with an electronic map, showing the known positions of 104 mounds of the Cahokia group. A yellow spoked circle shows the position of Woodhenge and Monk's Mound is encased within a slightly offset purple rectangle. The dimensions of that rectangle are based upon Reed's 1968 solid core survey to determine the exact (original or intended) design dimensions of Monk's Mound. Each outlying mound, standing at a measured distance and angle from the hub position atop Monk's Mound, was a classroom in its own right and contained very important codes of position, essential to the knowledge-base of students of navigation and cyclic astronomy, learning their craft at Cahokia.

The exact positions of mounds 1 & 2 have been determined after a very careful AutoCAD overdraw of the University of Wisconsin, Milwaukee map of mound positions. The process involves inclusion of roads, rivers, buildings and all prominent landmarks that appeared on the UWM map. After very careful scaling and marginal rotating of the electronic map in AutoCAD, it is then superimposed over the Google Earth image and tiny refinements made to ensure that everything is fitting perfectly.
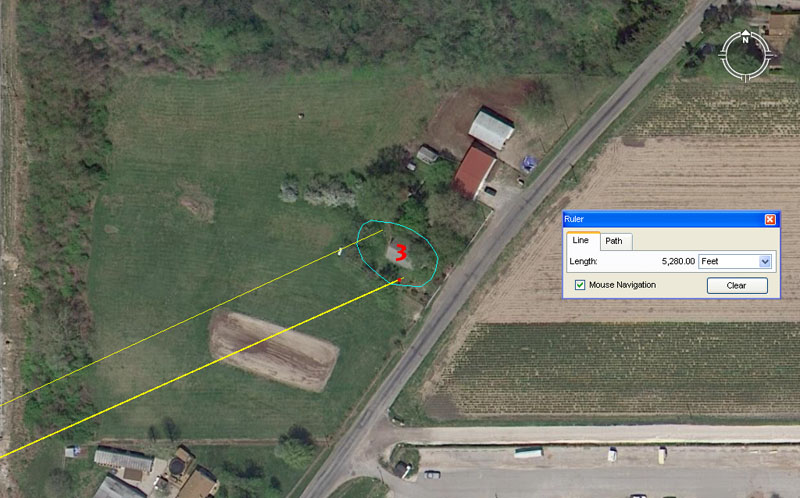
Mound 1. This mound has the distinction of being a due East marker for Monk's Mound. It sits at a coded distance of 6180.34' at an azimuth angle of 90-degrees.
It's obligatory that the 6180.34 (or a ratio expression of the same) value will be found at a site as immense and mathematically sophisticated as the Cahokia Mounds. The dynamic tutorial contained within this distance value relates to PHI and more especially to the PHI reciprocal, which ratios were essential knowledge to ancient civilisations and much used, for very practical applications, on a daily basis. Here's an example:
The American capacity for dry-goods called a "bushel" is a very ancient volume that has it's origins in Babylon and Britain. The ancient Sumerians-Babylonians had a volume called a Homer, which was exactly 21600 cubic inches. This survived in Britain as a lesser capacity "bushel", which was 2160 cubic inches or one tenth of a Homer. As the years wearied on and people (mostly because the unwelcome interference of the church and forced-Christianisatian) began to forget the significance and required precision of the old numbers, a bushel began to be described as "any round measure with a plain and even bottom, being 18.5 inches wide throughout and 8 inches deep". This description of a bushel tub, from the old English Winchester Standard, was later adopted by the new colony in North America and that is why the American bushel is the strange, shortfall value of 2150.42 cubic inches.
So what went wrong and caused the capacity to drift slightly off the highly factorable value of 2160 cubic inches?
All of the great cousin civilisations of antiquity used the PHI reciprocal formula in order to fabricate their "round" tubs of capacity used in the market place. Every volume within a tub had to carry codes that related to some aspect of ancient sciences. Each capacity, whether large or small, had to generate a number related to say, the cycle of the Moon or the Precession of the Equinoxes or, perhaps, the equatorial circumference of the Earth, etc. If everyone was using the numbers everyday, then they could never be lost.
The American bushel is supposed to relate to the duration of Precession of the Equinoxes. In the 25920-year cycle of Precession, the Sun spends 2160-years in each house of the Zodiac. The true (ancient) formula for building an American bushel barrel is:
10-inches ÷ 1.6180339 (PHI) = 6.18034". In order to then fabricate the flat, round base of the bushel tub one used 3 of these increments or 18.54102". So, the old English Winchester Standard had "drifted" and had been "rounded" downwards very slightly, but really needed that extra 1/25th of an inch in order achieve precise results.
Therefore: Old era carpenters would join 3 six inch planks together and then, using a string attached to a nail hammered in at the centre, draw a circle that was 18.54102" (very close to 187/13ths) in diameter. They would then cut the round base for their tub and, because they had used the PHI reciprocal as their formula, the base was exactly 270 square inches of surface area. They now added sides that extended exactly 8-inches above the flat base, giving a capacity of 270" X 8" = 2160 cubic inches.
The widespread use of PHI and especially the PHI reciprocal meant that there could be peace in the marketplaces of the ancient cousin nations and that there was no excuse for "short-measures", leading to disputes between grain vendors and purchasers. If a dispute arose, the capacity of the vendor's measuring tub could be checked by the marketplace judge and, in ancient times, anyone caught-out trying to defraud was generally dealt with very severely (in Scandinavian marketplaces it could mean the death penalty). It was essential to maintain stringent standards in weights, measures & volumes in order to maintain the public peace.
With this mound sitting exactly due east of the centre of Monk's Mound, navigational students atop Monk's mound could set the compass disk on their manual theodolites (alidade sighting rules) by this marker, then read the exact angle to any other target around the horizon.
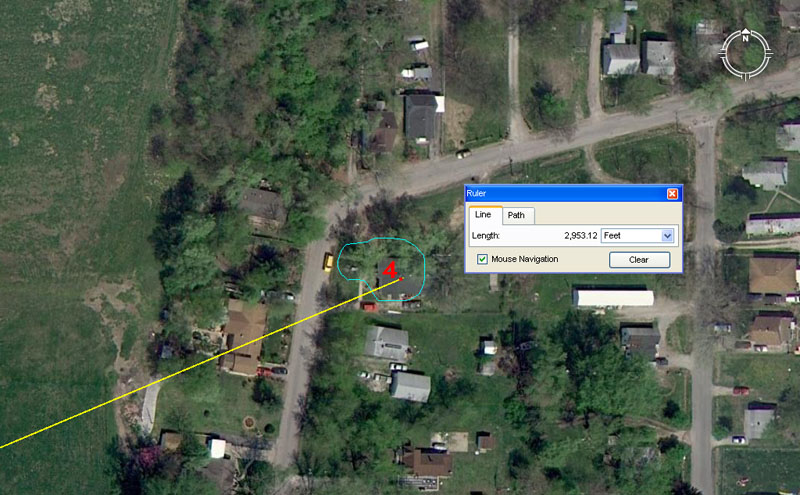
Mound 2. This mound is quite wrecked, but complies to a distance of 6075' from Monk's Mound centre @ an azimuth angle of 88-degrees. The distance onto the mound could likewise be read as 6048' (1-minute of equatorial arc under the "6 &7" navigational system using a mile of 5250') or 6050' (1-minute of equatorial arc under the "11" navigational system using a mile of 5280') and all of these tutorials would have been included.